
If two circles intersect at two points, prove that their center lies on the perpendicular bisector of the common chord.
Answer
475.2k+ views
Hint: For solving this question, we will first draw the diagram having two circles with center
(I) SSS congruence rule and CPCT (corresponding parts of congruent triangles)
(II) SAS congruence rule and CPCT (corresponding parts of congruent triangles).
(III) Linear pair: If the sum of angles is
(IV) Vertically opposite angles are always equal.
Complete step by step answer:
Let us first draw a diagram as per the question requires.
Let the two circles meet each other at two common points P and Q. Let the center of these circles be
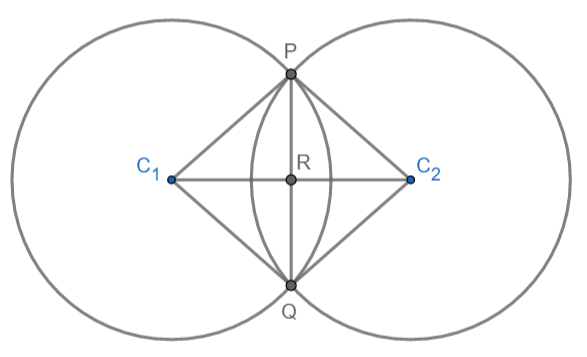
We need to prove that, center lies on the perpendicular bisector of the common chord. According to the diagram, we need to prove that PQ bisects
We have joined
In
We know that,
Similarly,
Also
Therefore, in both triangles all corresponding sides are equal.
So by SSS congruence rule,
By CPCT (corresponding parts of congruent triangles) we can say that
Now in
We know that
Therefore, by SAS congruence rule,
Therefore, PQ bisects OX.
Now we need to show if it bisects perpendicularly. From
As we can see PQ is a line so angles
So we can say that
From (2) we can say that
Dividing both sides by 2 we get
Hence,
Now if PQ and
Therefore,
Hence
Therefore
Hence
Hence proved.
Note: Students should keep in mind all the properties used for solving this sum. They should try to prove all four angles as
(I) SSS congruence rule and CPCT (corresponding parts of congruent triangles)
(II) SAS congruence rule and CPCT (corresponding parts of congruent triangles).
(III) Linear pair: If the sum of angles is
(IV) Vertically opposite angles are always equal.
Complete step by step answer:
Let us first draw a diagram as per the question requires.
Let the two circles meet each other at two common points P and Q. Let the center of these circles be

We need to prove that, center lies on the perpendicular bisector of the common chord. According to the diagram, we need to prove that PQ bisects
We have joined
In
We know that,
Similarly,
Also
Therefore, in both triangles all corresponding sides are equal.
So by SSS congruence rule,
By CPCT (corresponding parts of congruent triangles) we can say that
Now in
We know that
Therefore, by SAS congruence rule,
Therefore, PQ bisects OX.
Now we need to show if it bisects perpendicularly. From
As we can see PQ is a line so angles
So we can say that
From (2) we can say that
Dividing both sides by 2 we get
Hence,
Now if PQ and
Therefore,
Hence
Therefore
Hence
Hence proved.
Note: Students should keep in mind all the properties used for solving this sum. They should try to prove all four angles as
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




