
If x, y, z are three natural numbers in A.P. such that $x+y+z=30$ then the possible number of ordered triplet (x, y, z) is
A. 18
B. 19
C. 20
D. 21
Answer
522k+ views
Hint: Take the common difference as ‘d’. Substitute the values of x, y and z in the equation $x+y+z=30$. From the simplified equation, find the AP and substitute different values of d, which gives the total number of possibilities of ordered triplet.
Complete step-by-step answer:
It is said that x, y and z represent 3 natural numbers in AP.
AP represents Arithmetic progression. It is a sequence of numbers such that the difference between the consecutive terms is constant.
Difference here means the second minus the first term.
Let us consider ‘d’ as the common difference of the Arithmetic progression and ‘d’ is a natural number.
Here ‘x’ is the first term of AP.
y is the 2nd term of AP and ‘z’ the 3rd term.
Now, we need to find the difference between consecutive terms.
So, 2nd term – 1st term = d
$\Rightarrow y-x=d$
Rearrange the terms $x=y-d$.
Similarly, 3rd term – 2nd term = d.
$z-y=d\Rightarrow z=y+d$
Now, substitute the value of x and z in the equation $x+y+z=30$.
$\begin{align}
& \Rightarrow \left( y-d \right)+y+\left( y+d \right)=30 \\
& \Rightarrow y-d+y+y+d=30 \\
& \Rightarrow y+y+y=30 \\
& \Rightarrow 3y=30 \\
& \therefore y=\dfrac{30}{3}=10 \\
& \therefore x=y-d=10-d \\
& z=y+d=10+d \\
\end{align}$
$\therefore $ The AP of x, y, z is now equal to (10 – d), 10, (10 + d).
Now, let’s consider d =1, then AP becomes 9, 10, 11.
Similarly,
d = 2, AP = 8, 10, 12
d = 3, AP = 7, 10, 13
d = 4, AP = 6, 10, 14
d = 5, AP = 5, 10, 15
d = 6, AP = 4, 10, 16
d = 7, AP = 3, 10, 17
d = 8, AP = 2, 10, 18
d = 9, AP = 1, 10, 19
So these combinations of ordered triplets are possible.
i.e. now there are 9 possibilities which are the same for (19, 1), (18, 2)…..
$\therefore $ Number of possibilities $=9\times 2=18$ possibilities
Hence option A is the correct answer.
Note: The possibilities available can be represented as
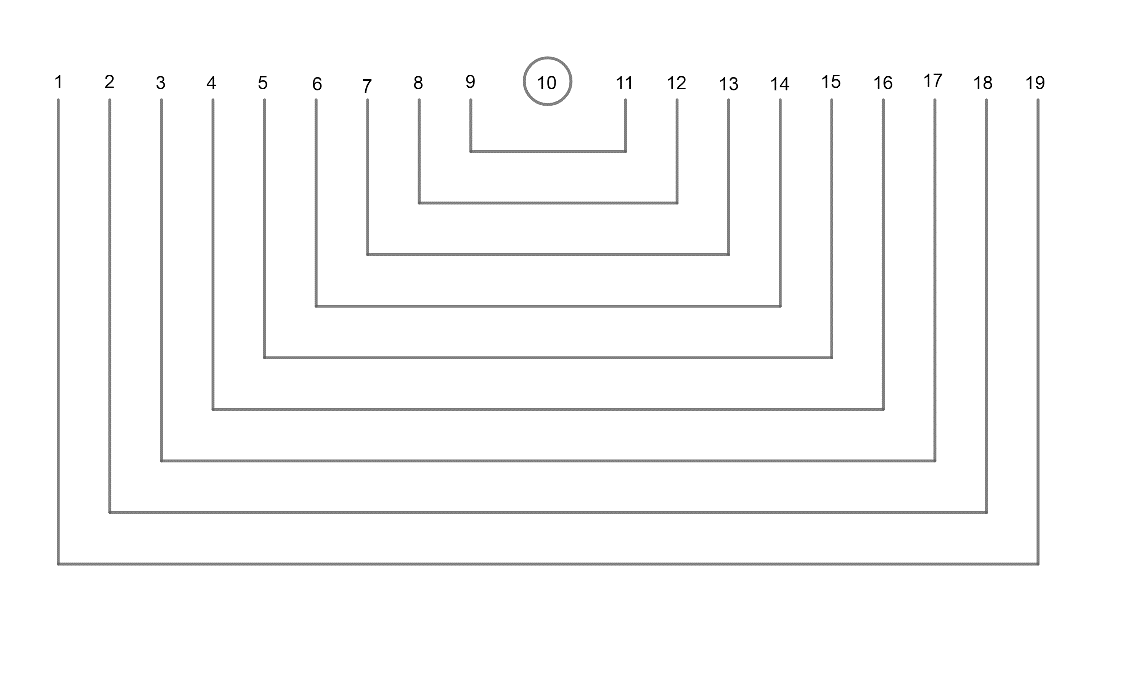
Complete step-by-step answer:
It is said that x, y and z represent 3 natural numbers in AP.
AP represents Arithmetic progression. It is a sequence of numbers such that the difference between the consecutive terms is constant.
Difference here means the second minus the first term.
Let us consider ‘d’ as the common difference of the Arithmetic progression and ‘d’ is a natural number.
Here ‘x’ is the first term of AP.
y is the 2nd term of AP and ‘z’ the 3rd term.
Now, we need to find the difference between consecutive terms.
So, 2nd term – 1st term = d
$\Rightarrow y-x=d$
Rearrange the terms $x=y-d$.
Similarly, 3rd term – 2nd term = d.
$z-y=d\Rightarrow z=y+d$
Now, substitute the value of x and z in the equation $x+y+z=30$.
$\begin{align}
& \Rightarrow \left( y-d \right)+y+\left( y+d \right)=30 \\
& \Rightarrow y-d+y+y+d=30 \\
& \Rightarrow y+y+y=30 \\
& \Rightarrow 3y=30 \\
& \therefore y=\dfrac{30}{3}=10 \\
& \therefore x=y-d=10-d \\
& z=y+d=10+d \\
\end{align}$
$\therefore $ The AP of x, y, z is now equal to (10 – d), 10, (10 + d).
Now, let’s consider d =1, then AP becomes 9, 10, 11.
Similarly,
d = 2, AP = 8, 10, 12
d = 3, AP = 7, 10, 13
d = 4, AP = 6, 10, 14
d = 5, AP = 5, 10, 15
d = 6, AP = 4, 10, 16
d = 7, AP = 3, 10, 17
d = 8, AP = 2, 10, 18
d = 9, AP = 1, 10, 19
So these combinations of ordered triplets are possible.
i.e. now there are 9 possibilities which are the same for (19, 1), (18, 2)…..
$\therefore $ Number of possibilities $=9\times 2=18$ possibilities
Hence option A is the correct answer.
Note: The possibilities available can be represented as

Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
Truly whole mankind is one was declared by the Kannada class 10 social science CBSE

Explain the three major features of the shiwaliks class 10 social science CBSE

Find the area of the minor segment of a circle of radius class 10 maths CBSE

Distinguish between the reserved forests and protected class 10 biology CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

A gulab jamun contains sugar syrup up to about 30 of class 10 maths CBSE




