Question

In a figure, a crescent is formed by two circles which touch at A. C is the centre of a larger circle. The width of the crescent at BD is 9 cm and at EF is 5cm. Find the radii of two circles.

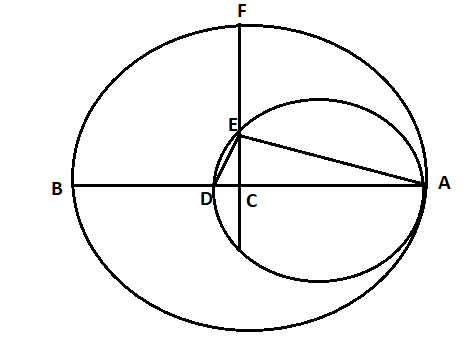
Answer 1

Hint: According to given in the question we have to determine the radii of two circles. When in a figure, a crescent is formed by two circles which touch at A. C is the centre of a larger circle. The width of the crescent at BD is 9 cm and at EF is 5cm. So first of all we have to let the radii of two circles. As mentioned in the question that the width of the crescent at BD is 9 cm and at EF is 5cm. So we have to determine the difference between the both of the radius we let.
Now we have to join line AE and DE and let the \[\angle CAE\] to determine the \[\angle AEC\] and now we have to consider the $\Delta ACE$ and $\Delta DCE$ to determine the \[\angle ECD\] and to make both of triangles congruent to each other.
Now we have to apply the congruent rule for both of the triangles $\Delta ACE$ and $\Delta ECD$. We can also understand this with the help of the diagram given below.

Formula used:
$ \Rightarrow {(a - b)^2} = {a^2} + {b^2} - 2ab$……………………………………(A)
Now we have to substitute all of the values to determine both of the radiuses.

Complete step by step answer:
Step 1: First of all we have to let the radius of both circles. So let the radius of the larger circle is R and let the radius of the smaller circle is r which we can understand with the help of the diagram as below:

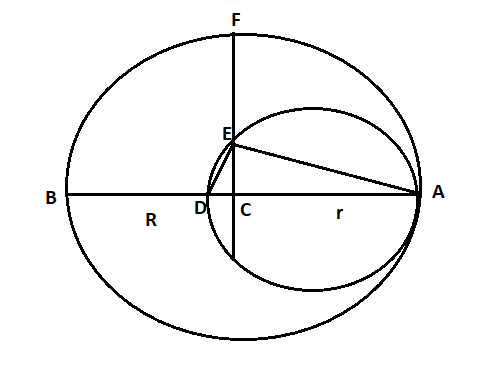
Step 2: Now as mentioned in the question the length of BD is 9cm so we can determine the difference between the diameters of the both circles.
$
   \Rightarrow 2R - 2r = 9 \\
   \Rightarrow R - r = \dfrac{9}{2} \\
   \Rightarrow R - r = 4.5........................(1) \\
 $
Step 3: Now we have to join the line AE and DE and we have to let the \[\angle CAE\] is $\theta $, to determine the \[\angle AEC\] we can also understand with the help of the diagram as given below,

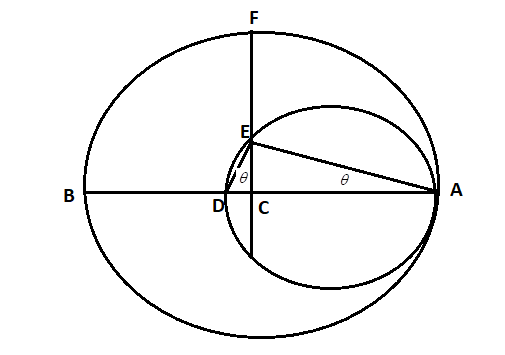
Step 4: Now we have to determine the \[\angle DEC\] with the help of $\angle AED$ and $\angle AEC$
$
   \Rightarrow \angle AED = {90^0} \\
   \Rightarrow \angle AED = \angle AEC + \angle DEC \\
 $
On substituting all the angles in the expression obtain just above,
$
   \Rightarrow \angle DEC = {90^0} - ({90^0} - \theta ) \\
   \Rightarrow \angle DEC = \theta \\
 $
Step 5: Now, we have to make the $\Delta ACE$ and $\Delta DCE$ congruent with the help of AA similarity criterion rule.
$ \Rightarrow \angle CAE = \angle CED = \theta $ and
$ \Rightarrow \angle ACE = \angle ECD = {90^0}$
Now, we can say that,
$ \Rightarrow \dfrac{{AC}}{{EC}} = \dfrac{{CE}}{{CD}}$………………………………..(2)
Step 6: Now, as we know that, $EC = CF - EF$ and $CD = BC - BD$. So, now substituting all the values in expression (2) as obtain in the solution step 5
$ \Rightarrow \dfrac{{AC}}{{CF - EF}} = \dfrac{{CF - EF}}{{BC - BD}}$
On substituting all the values in the expression above,
$
   \Rightarrow \dfrac{R}{{R - 5}} = \dfrac{{R - 5}}{{R - 9}} \\
   \Rightarrow R(R - 9) = (R - 5)(R - 5) \\
   \Rightarrow {R^2} - 9R = {(R - 5)^2} \\
 $
Now, to solve quadratic expression as obtained just above, we have to use the formula A as mentioned in the solution hint.
$
   \Rightarrow {R^2} - 9R = {R^2} + 25 - 10R \\
   \Rightarrow - 9R = 25 - 10R \\
   \Rightarrow - 9R + 10R = 25 \\
   \Rightarrow R = 25cm \\
 $
Step 7: On substituting this value of R in the expression (1) as obtain in the solution step (2)
$
   \Rightarrow 25 - r = 4.5 \\
   \Rightarrow 25 - 4.5 = r \\
   \Rightarrow r = 20.5cm \\
 $

Hence, as mentioned in the formula (A) as mentioned in the solution hint. We have determined both of the radiuses which are $R = 25cm$ and $r = 20.5cm$.

Note: It is necessary to make both of the triangles $\Delta ACE$ and $\Delta ECD$ with the help of the AA rule in which we have to make to angles similar to each other to make the triangles congruent to each other to determine the radiuses.
To find the radiuses we have to substitute the lines as $EC = CF - EF$ and $CD = BC - BD$ so that we can easily solve the expression obtained.