
In a Kundt’s tube experiment, the heaps of lycopodium powder are collected at 20cm separations. What will be the frequency of the tuning fork used?
Answer
492k+ views
Hint: The Kundt’s tube experiment is the acoustic experiment which describes the longitudinal wave pattern in the sound waves. The separation of piles formed by the given sample is a direct indicator of the characteristics of the sound wave used. The speed of sound in air is 340
Formula Used:
The basic formula used in a Kundt’s experiment is –
Where, c is the speed of sound in vacuum or air
Complete answer:
The Kundt’s tube experiment consists of a transparent horizontal tube with a small amount of the given sample, here it is the lycopodium. The one end of the tube has a single signal generator which can produce a sound wave of single frequency
The given figures give the amplitude variation at two instincts.
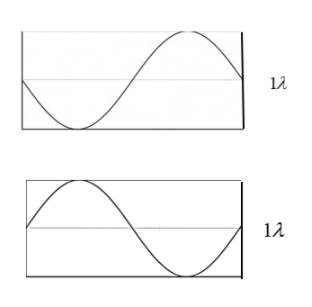
It is clear that the piston will be at equilibrium if
Also, we know from the experiment that the powder used has a tendency to pile at distance half of the wavelengths.
Now using this idea and given data, we can find the frequency of the sound used.
Now, using the basic formula relating speed, wavelength and frequency we can find the frequency of the sound.
The frequency with which the experiment was conducted is 850Hz.
Option B is the suitable answer.
Note:
In an experiment, the unknown we usually find is the wavelength of the generated sound wave. We find it similarly by measuring the distance between the piles of the lycopodium powder and applying the basic formula relating wavelength, frequency and speed of sound in the medium.
The experiment is also a proof for the longitudinal nature of the sound waves.
Formula Used:
The basic formula used in a Kundt’s experiment is –
Where, c is the speed of sound in vacuum or air
Complete answer:
The Kundt’s tube experiment consists of a transparent horizontal tube with a small amount of the given sample, here it is the lycopodium. The one end of the tube has a single signal generator which can produce a sound wave of single frequency
The given figures give the amplitude variation at two instincts.

It is clear that the piston will be at equilibrium if
Also, we know from the experiment that the powder used has a tendency to pile at distance half of the wavelengths.
Now using this idea and given data, we can find the frequency of the sound used.
Now, using the basic formula relating speed, wavelength and frequency we can find the frequency of the sound.
The frequency with which the experiment was conducted is 850Hz.
Option B is the suitable answer.
Note:
In an experiment, the unknown we usually find is the wavelength of the generated sound wave. We find it similarly by measuring the distance between the piles of the lycopodium powder and applying the basic formula relating wavelength, frequency and speed of sound in the medium.
The experiment is also a proof for the longitudinal nature of the sound waves.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




