Question

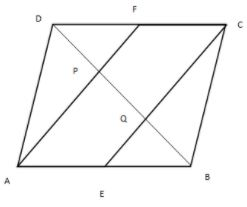
In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively (according to the figure). Show that the line segments AF and EC trisect the diagonal BD.

Answer 1

Hint: In order to show that the line segments AF and EC trisect the diagonal BD we use the Midpoint theorem and general properties of parallelogram.
Midpoint theorem states that the midpoint of a side in a parallelogram bisects it in two halves. Opposite sides of a parallelogram are parallel and equal in length.

Complete step-by-step answer:
Given that ABCD is a parallelogram.
Using the property of parallelogram, we say
AB || DC and AB = DC.

E is the midpoint of AB
${\text{AE = }}\dfrac{1}{2}{\text{AB - - - - - }}\left( 1 \right)$
Also F is the midpoint of CD
Therefore ${\text{CF = }}\dfrac{1}{2}{\text{CD}}$
${\text{CF = }}\dfrac{1}{2}{\text{AB }}\left( {\because {\text{CD = AB}}} \right){\text{ - - - - }}\left( 2 \right)$

From equation (1) and (2), we get
AE = CF
Also,
AE || CF (∵AB || DC)
Thus a pair of opposite sides of a quadrilateral AECF are parallel and equal.
Hence quadrilateral AECF is a parallelogram.
So, now we have
EC || AF
EQ || AP and QC || PF

In ∆BPA, E is the midpoint of BA and EQ || AP
Using mid-point theorem, we have
BQ || PQ ---- (3)
Similarly by taking ∆CQD, we can prove that
DP = QP ---- (4)
From (3) and (4), we get
BQ = QP = PD
Therefore, AF and CE trisect the diagonal BD.

Note: In order to solve this type of problems the key is to always remember that symmetry allows to reduce a theorem to a computation. Never try to solve a problem with a figure by solving directly without proof. In case of trisection, we have proved that all the three parts of the line are equal.