Question

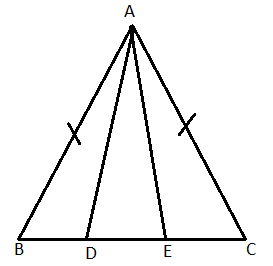
In an isosceles triangle ABC with AB = AC, D and E are points on BC such that BE = CD. Show that AD = AE.

Answer 1

Hint: Use the property given as “Angles of equal opposite sides are equal in a triangle”, to prove the triangles ABD and C.P.C.T prove the sides AD and AE are equal to each other.

Complete step-by-step answer:
As we have an isosceles triangle ABC with AB = AC, where D and E are points on BC such that BE = CD and we need to prove that the sides AD and AE are equal i.e. AD = AE.
So, diagram can be given as

So, we have
AB = AC……………..(i)
BE = CD…………….(ii)
Now, we know the property of triangles that opposite angles of the opposite sides are equal if sides are equal. It means \[\angle B\] and $\angle C$ will be equal as opposite sides of $\angle B$ and $\angle C$ i.e. AC and AB, are equal. Hence, we get
$\angle B=\angle C...............\left( iii \right)$
And from equation (ii), we (iii) have BE = CD
Now, subtract DE from both sides of terms of the above equation. So, we get
BE – DE = CD – DE
Now, we can observe that the diagram is replaced by side BD and CD – DE by side CE. Hence, we get above equation as
BD = CE………………….(iv)
Now, in $\Delta ABD$and $\Delta AEC$ , we have
AB = AC (from equation (i))
\[\angle B=\angle C\] (from equation (iii))
BD = CE (from equation (iv))
Hence, $\Delta ABD$ is congruent to $\Delta AEC$ by SAS criteria of congruence. So, we get
$\Delta ABD\cong \Delta AEC$
So, now all the corresponding sides and angles of triangles ABD and ACE are equal by the C.P.C.T property of congruent triangles.
Hence, we get
AD = AE ( C.P.C.T)
So, it proved that AD and AE are equal.


Note: Another approach for proving AD = AE, we can prove the triangles ABE and ADE as congruent triangle in the following way:
AB = AC
BE = DC
$\angle B=\angle C$
By SAS criteria $\Delta ABE\cong \Delta ADC.$So, it can be another approach. Getting the equation $\angle B=\angle C$ is the key point for proving the triangles ABD and ACE to congruent problems and need to use property for getting it.