
In

Answer
393k+ views
Hint: In a right-angled triangle the sine of an angle is the length of the opposite side of an angle divided by the hypotenuse of the triangle. Here we need to calculate the hypotenuse of the triangle. So we should use this formula to calculate the hypotenuse by replacing the sine value and length of the opposite side of the angle. From the trigonometric table, we know that
Complete step by step solution:
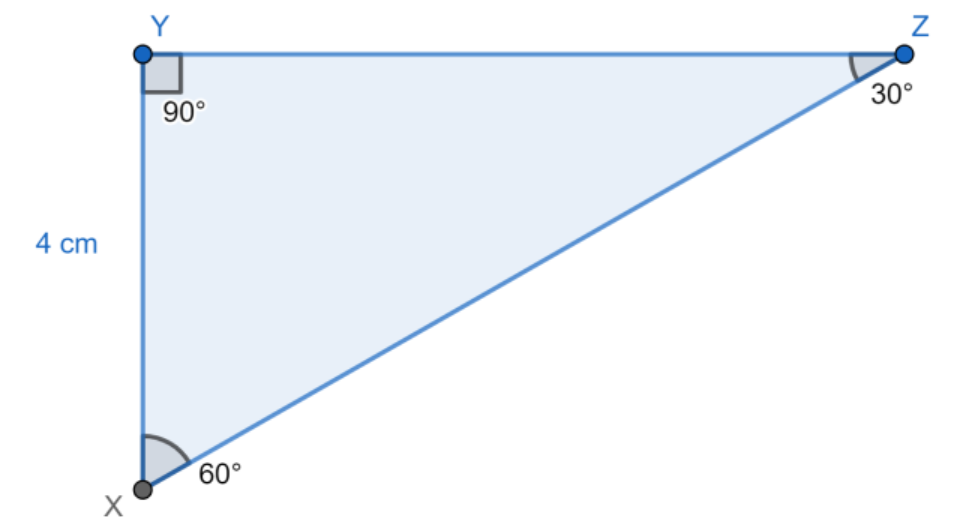
From the figure, we can see
Here,
We need to find the value of
Here the base of the triangle is
First, we calculate the sine value of
According to the formula of sine of an angle we have:
Taking the unknown value i.e. side
Replacing the required values in the above formula we get:
Hence, the required value of the side
Note: One should be well acquainted with the trigonometric table. Calculating the sine of the angle is very important over here. Much redundant information is also given. So choosing the correct information i.e. which angle to use to find the unknown side is very crucial. This method is useful only for right-angled triangles.
Complete step by step solution:
From the figure, we can see
Here,
We need to find the value of
Here the base of the triangle is
First, we calculate the sine value of
According to the formula of sine of an angle we have:
Taking the unknown value i.e. side
Replacing the required values in the above formula we get:
Hence, the required value of the side
Note: One should be well acquainted with the trigonometric table. Calculating the sine of the angle is very important over here. Much redundant information is also given. So choosing the correct information i.e. which angle to use to find the unknown side is very crucial. This method is useful only for right-angled triangles.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




