
In diammonium hydrogen phosphate,
(This question has multiple correct options.)
(A)
(B)
(C) P is maximum
(D) N is minimum
Answer
441.3k+ views
Hint: To solve this question we first need to determine the molecular mass of diammonium hydrogen phosphate,
Complete answer:
Now, we know that the atomic mass of
N = 14.01 u
H = 1.010 u
P = 30.97 u
O = 16.00 u
Diammonium hydrogen phosphate,
So, the molar mass of diammonium hydrogen phosphate (
Now, the formula to calculate the percentage composition of a constituent in a compound is given by:
So, the percentage compositions of elements N, H, O, and P are as follows
From the above calculations, we can see that the percentage compositions of elements in
N = 21.21%
H = 6.870%
O = 48.46%
P = 23.45 %
Now, diammonium hydrogen phosphate is produced as follows
Hence, we can say that one mole of diammonium hydrogen phosphate (
Also, it can be said that one mole of diammonium hydrogen phosphate (
So, the percentage compositions of
From the above calculations, we can see that the percentage compositions of
So, the correct answers are option (A) and option (B).
Note:
It should be noted that upon dissociation at a temperature of
The structure of diammonium hydrogen phosphate salt is as follows.
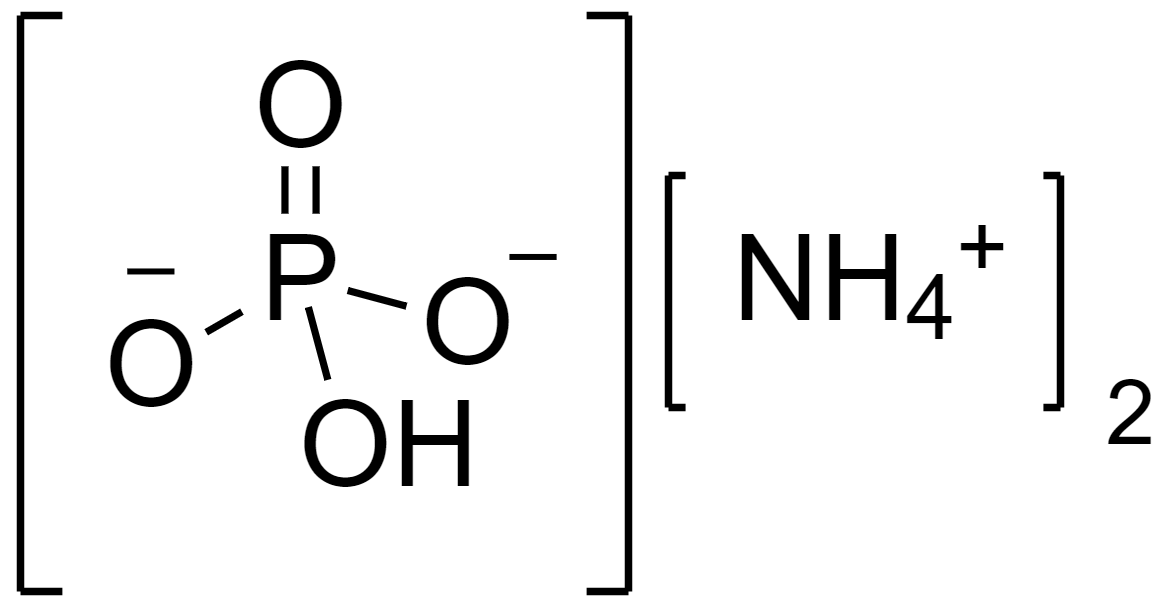
Complete answer:
Now, we know that the atomic mass of
N = 14.01 u
H = 1.010 u
P = 30.97 u
O = 16.00 u
Diammonium hydrogen phosphate,
So, the molar mass of diammonium hydrogen phosphate (
Now, the formula to calculate the percentage composition of a constituent in a compound is given by:
So, the percentage compositions of elements N, H, O, and P are as follows
From the above calculations, we can see that the percentage compositions of elements in
N = 21.21%
H = 6.870%
O = 48.46%
P = 23.45 %
Now, diammonium hydrogen phosphate is produced as follows
Hence, we can say that one mole of diammonium hydrogen phosphate (
Also, it can be said that one mole of diammonium hydrogen phosphate (
So, the percentage compositions of
From the above calculations, we can see that the percentage compositions of
So, the correct answers are option (A) and option (B).
Note:
It should be noted that upon dissociation at a temperature of
The structure of diammonium hydrogen phosphate salt is as follows.

Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

The reason why India adopted the policy of nonalignment class 11 social science CBSE

The plastids which are coloured green and colourless class 11 biology CBSE

Earth rotates in which direction A East to west B West class 11 physics CBSE

a Green plastids are called b Plant cell has a big class 11 biology CBSE




