
In fig. PQRS is a quadrilateral and T and U are respectively points on PS and RS such that
Prove that
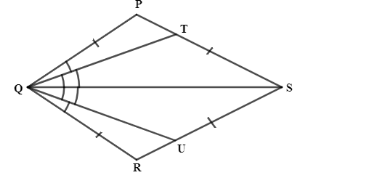

Answer
511.8k+ views
Hint: First of all, we will find the sum of angles of a quadrilateral and then using that we will find the triangles which are equivalent to each other. Then using the laws of triangles which is given as when two sides and one angle of two triangles are the same i.e. side angle side rule (SAS), then those two triangles are equivalent to each other. By using this, we will prove
Complete step-by-step answer:
Now, in question we are given that,
Now, on adding the terms of expression (i) and (ii) we will get,
Now, from the figure it can be seen that ,
And
Now, substituting the values of equation (iv) and (v) in expression (iii), we will get,
Now, we can see that there are two triangles in the quadrilateral PQRS, which are,
Now, in
And two angles are equal which we found in expression (iv) i.e.
We are also given that
Now, as two triangles of a quadrilateral are equivalent to each other, two opposite angles will also be equal, which can be given as,
Now, on observing the figure it can be seen that
Now, in
Two sides are same i.e.
And two angles are equal which we found in expression (ii) i.e.
We are also given that
So, from all these relations we can say that it satisfies the angle side angle theorem, which states that in triangle when, two angles and one side are common then those two triangles are equivalent to them, which can be given mathematically as,
Now, as two triangles of a quadrilateral are equivalent to each other, two opposite sides will also be equal, which can be given as,
Hence, proved as given in the question.
Note: In such a type of question, students might make mistakes in considering the same angle and same sides of the triangle and they might get confused in considering the equivalency of the triangles and due to that the sum might get wrong results, students must be careful in selecting corresponding sides of the two triangles equivalent to each other..
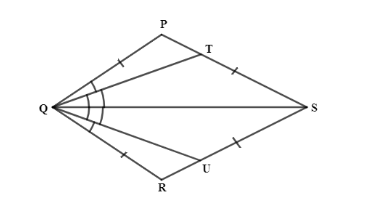
Complete step-by-step answer:
Now, in question we are given that,

Now, on adding the terms of expression (i) and (ii) we will get,
Now, from the figure it can be seen that ,
And
Now, substituting the values of equation (iv) and (v) in expression (iii), we will get,
Now, we can see that there are two triangles in the quadrilateral PQRS, which are,
Now, in
And two angles are equal which we found in expression (iv) i.e.
We are also given that
Now, as two triangles of a quadrilateral are equivalent to each other, two opposite angles will also be equal, which can be given as,
Now, on observing the figure it can be seen that
Now, in
Two sides are same i.e.
And two angles are equal which we found in expression (ii) i.e.
We are also given that
So, from all these relations we can say that it satisfies the angle side angle theorem, which states that in triangle when, two angles and one side are common then those two triangles are equivalent to them, which can be given mathematically as,
Now, as two triangles of a quadrilateral are equivalent to each other, two opposite sides will also be equal, which can be given as,
Hence, proved as given in the question.
Note: In such a type of question, students might make mistakes in considering the same angle and same sides of the triangle and they might get confused in considering the equivalency of the triangles and due to that the sum might get wrong results, students must be careful in selecting corresponding sides of the two triangles equivalent to each other..
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
What is the difference between Atleast and Atmost in class 9 maths CBSE

What was the capital of the king Kharavela of Kalinga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Describe the factors why Mumbai is called the Manchester class 9 social science CBSE

Write an essay in about 150 200 words on the following class 9 english CBSE

Write the 6 fundamental rights of India and explain in detail




