Question

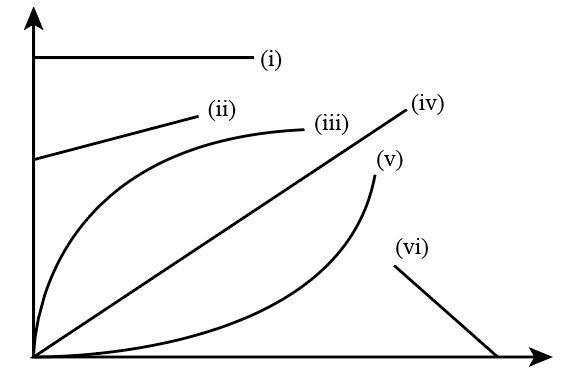
In figure shows the velocity-time graph for various situations. What does each graph indicate?

Answer 1

Hint: We are given with the velocity-time graph of a body for various conditions. Velocity is represented on the y-axis, and time is represented on the x-axis. We will closely observe each slope one by one to find the relationship between velocity and time.

Complete step by step answer:
From the slope of equation (i), we see that the velocity is not changing with the time, that means it is constant. From the slope of equation (i) we can write:
\[V = c\]
Here V is the velocity of the given body and c is a constant.

From equation (ii), we see that at the start of the slope, there is some velocity attained by the object and after that velocity is increasing linearly with time. Therefore, the object has some initial velocity which is independent of time so we can write:
\[V = {\rm{a}}t + {\rm{b}}\]
Here a and b are constant values, and t is time.

From the slope of equation (iii), we can conclude that the velocity of the given object is a square root function of time so we can write the equation for this situation as below:
\[V = {\rm{a}}\sqrt t \]

On observing the slope of equation (iv), we find that the velocity is increasing linearly with the time, that means it has a linear relationship with time which can be expressed as:
\[V = {\rm{a}}t\]

From the slope of equation (v), we can write that the velocity is a square function of time, or we can say it is increasing with time in a quadratic form.
\[V \propto {t^2}\]

On closely observing the slope of equation (vi), we can see that velocity has some initial fixed value then it is decreasing with time by following a linear relationship so we can write:
\[V = {\rm{a}} - {\rm{b}}t\]

Note:
We can remember that if the slope of a body on the velocity-time graph is following a downward concave path, that means velocity is a square root function of time and if the slope is concave upward, velocity is in quadratic relationship with time.