
In LCR circuit, the power factor at resonance is
A. Less than one
B. Greater than one
C. Unity
D. Can’t predict

Answer
502.5k+ views
Hint: For an RLC circuit in series, consider the same current flowing through the circuit and find out the voltages across the inductor, capacitor and resistor. Apply the condition of resonance to find the value of the phase difference in the voltage and current. Then apply it in the power factor formula.
Formula used: The condition for resonance in an RLC series circuit:
Formula for real power:
Complete step-by-step answer:
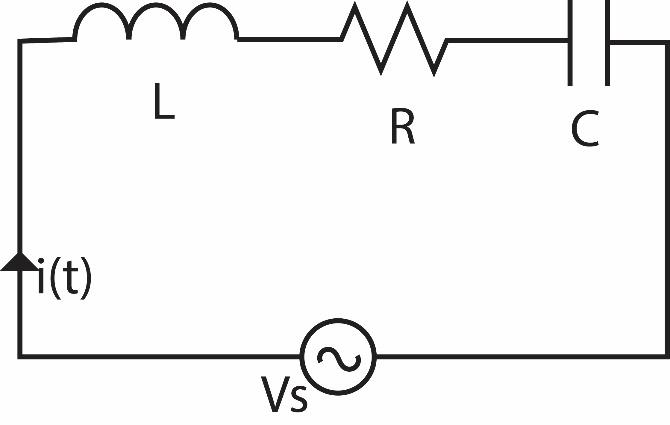
For the LCR circuit given in the figure, when the input is a sinusoidal wave, the following analysis can be proposed.
The voltage across the resistor remains in phase with the current in the circuit. The voltage across the inductor leads the current by
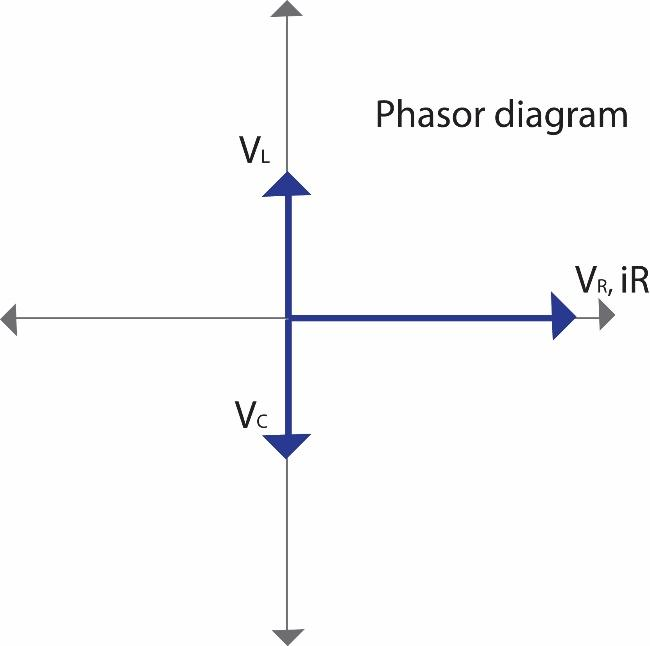
Where, for capacitor:
For inductor:
For resistor:
For the circuit to be resonant,
That is the net voltage and the current are in the same phase.
Suppose the instantaneous current through a circuit is
Where,
Power factor is defined as the ratio of the actual electric power dissipated by AC circuit to the product of the rms (root mean square) voltage and current. The non-useful work is the contribution of the reactance.
Since, for a circuit operating at resonance, the phase difference between voltage and current is zero. Therefore, the power factor becomes
The answer to this question is 1.
Note: At resonance in a circuit, the frequency of the source helps to balance the reactive elements in the circuit. The capacitive and the inductive load balance each other out. And the resultant impedance is only resistive in nature. Thus, all the work done is the real work. Hence, by the definition of power factor, it becomes 1.
Formula used: The condition for resonance in an RLC series circuit:
Formula for real power:
Complete step-by-step answer:
For the LCR circuit given in the figure, when the input is a sinusoidal wave, the following analysis can be proposed.
The voltage across the resistor remains in phase with the current in the circuit. The voltage across the inductor leads the current by

Where, for capacitor:
For inductor:
For resistor:
For the circuit to be resonant,
That is the net voltage and the current are in the same phase.
Suppose the instantaneous current through a circuit is
Where,
Power factor is defined as the ratio of the actual electric power dissipated by AC circuit to the product of the rms (root mean square) voltage and current. The non-useful work is the contribution of the reactance.
Since, for a circuit operating at resonance, the phase difference between voltage and current is zero. Therefore, the power factor becomes
The answer to this question is 1.
Note: At resonance in a circuit, the frequency of the source helps to balance the reactive elements in the circuit. The capacitive and the inductive load balance each other out. And the resultant impedance is only resistive in nature. Thus, all the work done is the real work. Hence, by the definition of power factor, it becomes 1.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




