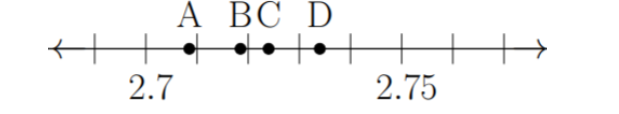Answer
384.6k+ views
Hint: A set is a collection of objects, typically grouped within braces \[\{ \} \], where each object is called an element or digit. For example, {apple, mango, banana} is a set of fruits. A subset is a set consisting of elements that belong to a given set. For example, {apple} is a subset of the set above.
Complete step-by-step answer:
Irrational numbers are defined as any number that cannot be written as a ratio of two integers. Simply, non terminating decimals that do not repeat are irrational. For example, \[\pi = 3.14159...\] and \[\sqrt 2 = 1.41421...\]
We have to find the letter whose value is near or approximately equal to \[e\] in the number line. First find the value of each part in the given number line by dividing the difference between the value and the number of parts.
A number line allows us to visually display real numbers by associating them with unique points on a line. The real number associated with a point is called a coordinate. A point on the real number line that is associated with a coordinate is called its graph.
To construct a number line, draw a horizontal line with arrows on both ends to indicate that it continues without bound. Next, choose any point to represent the number zero; this point is called the origin. Mark off consistent lengths on both sides of the origin and label each tick mark to define the scale. Positive real numbers lie to the right of the origin and negative real numbers lie to the left. Typically, each tick represents one unit.

Since the part between \[2.7\] and \[2.75\] is divided into \[5\] parts. So, each part measures
\[\dfrac{{2.75 - 2.7}}{5} = 0.01\].
\[e = 2.718\] is to be located on the number line.
So, on analysis, we can say that point \[B\] is the correct point.
So, the correct answer is “Option B”.
Note: Note that the scale need not always be one unit. Also note that the number zero \[(0)\] is neither positive nor negative. A number line is also known as a real number line. The number \[e\] is the base of the Natural Logarithms.
Complete step-by-step answer:
Irrational numbers are defined as any number that cannot be written as a ratio of two integers. Simply, non terminating decimals that do not repeat are irrational. For example, \[\pi = 3.14159...\] and \[\sqrt 2 = 1.41421...\]
We have to find the letter whose value is near or approximately equal to \[e\] in the number line. First find the value of each part in the given number line by dividing the difference between the value and the number of parts.
A number line allows us to visually display real numbers by associating them with unique points on a line. The real number associated with a point is called a coordinate. A point on the real number line that is associated with a coordinate is called its graph.
To construct a number line, draw a horizontal line with arrows on both ends to indicate that it continues without bound. Next, choose any point to represent the number zero; this point is called the origin. Mark off consistent lengths on both sides of the origin and label each tick mark to define the scale. Positive real numbers lie to the right of the origin and negative real numbers lie to the left. Typically, each tick represents one unit.

Since the part between \[2.7\] and \[2.75\] is divided into \[5\] parts. So, each part measures
\[\dfrac{{2.75 - 2.7}}{5} = 0.01\].
\[e = 2.718\] is to be located on the number line.
So, on analysis, we can say that point \[B\] is the correct point.
So, the correct answer is “Option B”.
Note: Note that the scale need not always be one unit. Also note that the number zero \[(0)\] is neither positive nor negative. A number line is also known as a real number line. The number \[e\] is the base of the Natural Logarithms.
Recently Updated Pages
The radius of curvature of a plane mirror is a positive class 10 physics CBSE

Choose the word which is closest to the opposite in class 10 english CBSE

Select the antonym for the following word from the class 10 english CBSE

Select the synonym for the given word Transparency class 10 english CBSE

Select the given word which means the opposite of the class 10 english CBSE

The purest form of carbon is a Graphite b Diamond c class 10 chemistry CBSE

Trending doubts
How do you graph the function fx 4x class 9 maths CBSE

Which are the Top 10 Largest Countries of the World?

Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

What is the meaning of sol in chemistry class 11 chemistry CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

The capital of British India was transferred from Calcutta class 10 social science CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

Capital of the Cheras was A Madurai B Muziri C Uraiyur class 10 social science CBSE

What organs are located on the left side of your body class 11 biology CBSE