
in the case of a simple pendulum, a graph has been represented between its kinetic energy, KE and its potential energy, PE against displacement
A.
B.
C.
D.




Answer
483k+ views
Hint: The kinetic energy in a simple harmonic motion can be found by taking the half of the product of the mass and square of the velocity. Using this find out the variation in potential energy and kinetic energy with a displacement. This will help you in answering this question.
Complete answer:
The kinetic energy in a simple harmonic motion can be found by taking the half of the product of the mass and square of the velocity. That is we can write that,
Where
Substituting this in the equation of kinetic energy will give,
As we all know the spring constant can be found as,
Substituting this value in the equation will give,
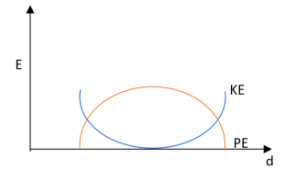
For a simple pendulum, the variation in kinetic energy and potential energy with displacement
If the displacement is zero, then,
If the displacement be,
Then we can write that,
Using these relations, we can plot the graph. That is the graph will look like this,
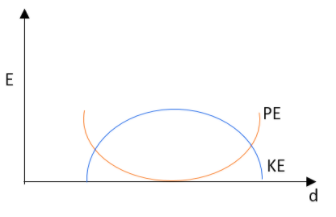
Therefore the correct answer has been obtained as option B.
Note:
The potential energy of a system is defined as the energy acquired by the system because of its orientation and position in the space. The kinetic energy of a system is defined as the energy possessed by a body because of the motion of the system. Both of them are having the same units of energy.
Complete answer:
The kinetic energy in a simple harmonic motion can be found by taking the half of the product of the mass and square of the velocity. That is we can write that,
Where
Substituting this in the equation of kinetic energy will give,
As we all know the spring constant can be found as,
Substituting this value in the equation will give,
For a simple pendulum, the variation in kinetic energy and potential energy with displacement
If the displacement is zero, then,
If the displacement be,
Then we can write that,
Using these relations, we can plot the graph. That is the graph will look like this,

Therefore the correct answer has been obtained as option B.
Note:
The potential energy of a system is defined as the energy acquired by the system because of its orientation and position in the space. The kinetic energy of a system is defined as the energy possessed by a body because of the motion of the system. Both of them are having the same units of energy.
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




