
In the centre of a rectangular plot of land of dimensions
Answer
500.1k+ views
Hint: Here, we need to find the dimensions of the area to be planted. We will assume the distance between the sides of the rectangular plot, and the sides of the rectangular portion to be covered by trees remains the same as
Complete step by step solution:
The trees are planted in the centre of the rectangular plot.
The distance between the sides of the rectangular plot, and the sides of the rectangular portion to be covered by trees remains the same.
Let the distance between the sides of the rectangular plot, and the sides of the rectangular portion to be covered by trees remains the same be
First, we will draw the diagram for this question.
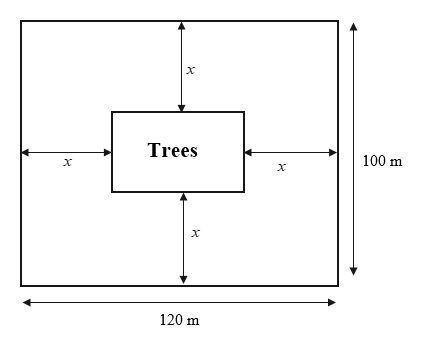
Here, we can observe that the rectangular portion to be covered by trees is equidistant from the sides of the rectangular plot. The distance is
Now, the area of a rectangle is the product of its length and breadth.
Therefore, we can find the area of the rectangular plot.
Substituting
Next, we know that the area of the plot not covered by trees is
We can find the area to be planted by subtracting the area of the plot not covered from trees from the total area of the rectangular plot.
Therefore, we get the area to be planted as
Now, from the diagram, we can find the length and breadth of the area to be planted.
Therefore, we get the area to be planted as
Substituting the area to be planted as
Simplifying the equation, we get
This is a quadratic equation. We will factorise the equation to get the value of
Factoring the quadratic equation, we get
Therefore, either
However, if
This is not possible as length cannot be negative.
Therefore, we get the value of
Substituting 35 for
Therefore, the dimensions of the area to be planted are
Additional Information
The social act of planting trees is being discussed here. This is also known as afforestation.
Planting trees is good for the environment.
Trees help in converting the carbon dioxide in the air to oxygen, and thus refreshing the air for us to breathe. This exchange of gases is important for us to survive.
Trees also give us various foods and products like resin.
Planting trees results in better quality of air, and prevents soil erosion.
Various wildlife animals also depend on trees for their survival.
Note:
We need to understand that we need to find the dimensions of the rectangular portion, not the area. We will make a mistake if we leave the answer at
Complete step by step solution:
The trees are planted in the centre of the rectangular plot.
The distance between the sides of the rectangular plot, and the sides of the rectangular portion to be covered by trees remains the same.
Let the distance between the sides of the rectangular plot, and the sides of the rectangular portion to be covered by trees remains the same be
First, we will draw the diagram for this question.

Here, we can observe that the rectangular portion to be covered by trees is equidistant from the sides of the rectangular plot. The distance is
Now, the area of a rectangle is the product of its length and breadth.
Therefore, we can find the area of the rectangular plot.
Substituting
Next, we know that the area of the plot not covered by trees is
We can find the area to be planted by subtracting the area of the plot not covered from trees from the total area of the rectangular plot.
Therefore, we get the area to be planted as
Now, from the diagram, we can find the length and breadth of the area to be planted.
Therefore, we get the area to be planted as
Substituting the area to be planted as
Simplifying the equation, we get
This is a quadratic equation. We will factorise the equation to get the value of
Factoring the quadratic equation, we get
Therefore, either
However, if
This is not possible as length cannot be negative.
Therefore, we get the value of
Substituting 35 for
Therefore, the dimensions of the area to be planted are
Additional Information
The social act of planting trees is being discussed here. This is also known as afforestation.
Planting trees is good for the environment.
Trees help in converting the carbon dioxide in the air to oxygen, and thus refreshing the air for us to breathe. This exchange of gases is important for us to survive.
Trees also give us various foods and products like resin.
Planting trees results in better quality of air, and prevents soil erosion.
Various wildlife animals also depend on trees for their survival.
Note:
We need to understand that we need to find the dimensions of the rectangular portion, not the area. We will make a mistake if we leave the answer at
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




