
In the figure above, find tan
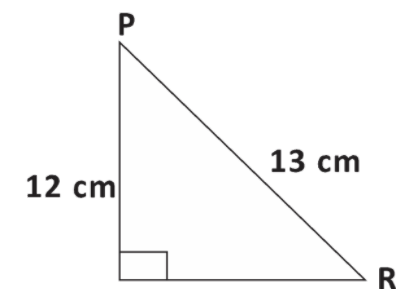
(A) 5
(B)
(C) 0
(D) none of these

Answer
493.5k+ views
Hint: To solve this question, we must have an idea about Pythagoras theorem for right-angled triangles.
Here the above picture is a right-angled triangle.
The sides of right-angled triangle named as base, perpendicular, and hypotenuse
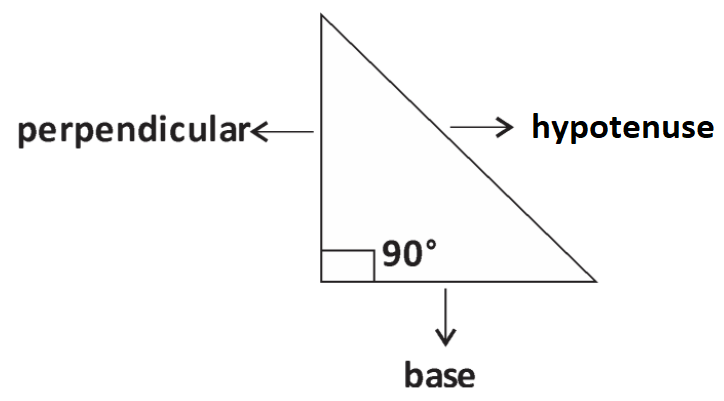
According to Pythagoras theorem,
Then,
Complete step by step answer:
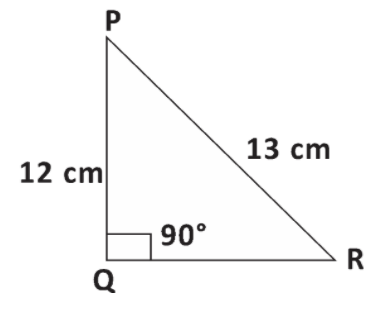
Given that
At first, we have to find out
Here,
By Pythagoras theorem,
Now, we calculate the value of tan p & cot R.
[for angle P, the opposite side of angle is perpendicular
Note: A right-angled triangle’s base is one of the sides that adjoins the 90-degree angle.
- The three main functions in trigonometry are sine, cosine, and tangent. It
Here the above picture is a right-angled triangle.
The sides of right-angled triangle named as base, perpendicular, and hypotenuse

According to Pythagoras theorem,
Then,
Complete step by step answer:

Given that
At first, we have to find out
Here,
By Pythagoras theorem,
Now, we calculate the value of tan p & cot R.
[for angle P, the opposite side of angle is perpendicular
Note: A right-angled triangle’s base is one of the sides that adjoins the 90-degree angle.
- The three main functions in trigonometry are sine, cosine, and tangent. It
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




