
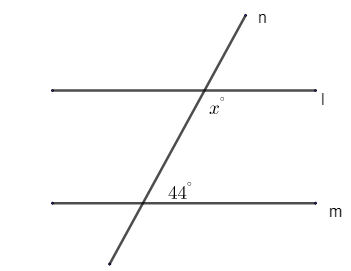
In the figure, find the value of x for which the lines l and m are parallel.
(a)
(b)
(c)
(d)

Answer
515.1k+ views
Hint: In the parallel lines use the concept of interior angles and apply the property that the sum of interior angles is
Complete step-by-step answer:
In the question we are given a figure and we have to find the value of x.
The figure is,
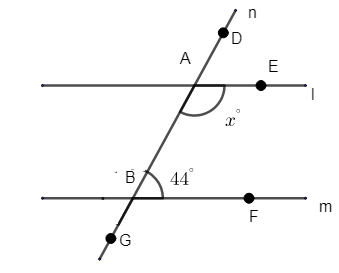
It contains two straight lines l, m which are parallel to each other which is cut by a straight line n or a transversal at two points let it be named as A, B.
So, here angle of A is measured as x and angle of B is given as B. As we know that here angle A and angle B together known as co – interior angles or we can say that if two parallel lines are cut by transversal like l and m by line n then
So, according to that,
As we are given that
Or,
So, the value of x is
Hence, the correct option is (a).
Note: We can also use the concept of corresponding angles, that corresponding angles are equal. Hence, corresponding angles are
Complete step-by-step answer:
In the question we are given a figure and we have to find the value of x.
The figure is,

It contains two straight lines l, m which are parallel to each other which is cut by a straight line n or a transversal at two points let it be named as A, B.
So, here angle of A is measured as x and angle of B is given as B. As we know that here angle A and angle B together known as co – interior angles or we can say that if two parallel lines are cut by transversal like l and m by line n then
So, according to that,
As we are given that
Or,
So, the value of x is
Hence, the correct option is (a).
Note: We can also use the concept of corresponding angles, that corresponding angles are equal. Hence, corresponding angles are
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE





