
In the figure, find the values of
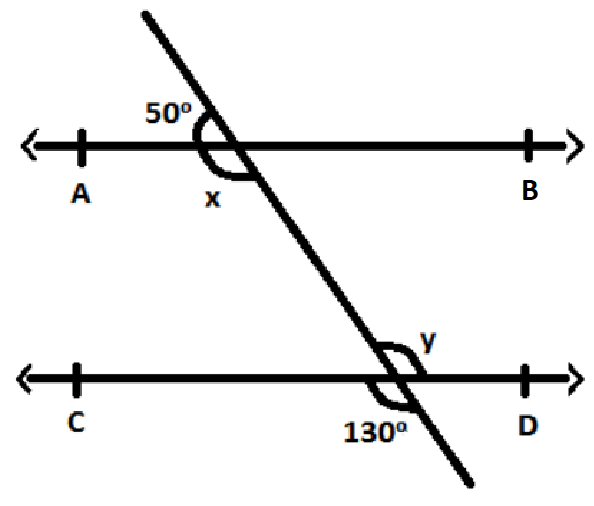

Answer
475.2k+ views
Hint: In this question, we need to determine the values of
Complete step by step answer:
In the given figure, a transversal intersects two lines
We know that the sum of linear pairs of angles is equal to
Therefore, we have,
We know that vertically opposite angles are equal. Here,
Therefore, we have,
Hence,
Therefore, alternate interior angles are equal.
From the theorem we know that if a transversal intersects two lines such that the pair of alternate interior angles are equal, then the lines are parallel.
Hence,
Note: In the question it is important to note that, the linear pair is a pair of angles that share a side and a base. Alternately, they are the two angles created along the line when two lines intersect. Here, the angle
Complete step by step answer:
In the given figure, a transversal intersects two lines
We know that the sum of linear pairs of angles is equal to
Therefore, we have,
We know that vertically opposite angles are equal. Here,
Therefore, we have,
Hence,
Therefore, alternate interior angles are equal.
From the theorem we know that if a transversal intersects two lines such that the pair of alternate interior angles are equal, then the lines are parallel.
Hence,
Note: In the question it is important to note that, the linear pair is a pair of angles that share a side and a base. Alternately, they are the two angles created along the line when two lines intersect. Here, the angle
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Give me the opposite gender of Duck class 8 english CBSE

Advantages and disadvantages of science




