
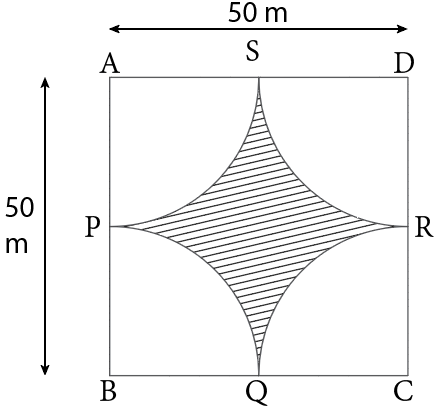
In the figure given here

Answer
495.6k+ views
Hint: Firstly, find the area of square
Then, find radii of the given quarter circles and thus find the total area of the 4 quarter circles.
Finally, subtract the area of 4 quarter circles from the area of the square to get the area of the required shaded area.
Complete step-by-step answer:
Here,
So the area of square
Now, there are 4 quarter circles given in the diagram with centres of the circles as points A, B, C and D and also points P, Q, R and S are the mid-points of side AB, BC, CD and AD respectively.
Thus, the length of the segment AP = PB = BQ = QC = CR = RD = DS = SA
So, the radii of the given quarter circles are 25 m each.
Now, the area of 4 quarter circles with radii 25 m each is given by
Thus, the are of given 4 quarter circles
Now, the area of the shaded part can be given by the difference of area of square and the area of 4 quarter circles.
Thus, we get the required area as
Note: Try to remember the following formula for solving these types of questions 1.Area of a square with length of side
2.Area of a circle with radius
3.Area of semicircle with radius of circle
4.Area of quarter circle with radius of circle
Then, find radii of the given quarter circles and thus find the total area of the 4 quarter circles.
Finally, subtract the area of 4 quarter circles from the area of the square to get the area of the required shaded area.
Complete step-by-step answer:
Here,
So the area of square
Now, there are 4 quarter circles given in the diagram with centres of the circles as points A, B, C and D and also points P, Q, R and S are the mid-points of side AB, BC, CD and AD respectively.
Thus, the length of the segment AP = PB = BQ = QC = CR = RD = DS = SA
So, the radii of the given quarter circles are 25 m each.
Now, the area of 4 quarter circles with radii 25 m each is given by
Thus, the are of given 4 quarter circles
Now, the area of the shaded part can be given by the difference of area of square and the area of 4 quarter circles.
Thus, we get the required area as
Note: Try to remember the following formula for solving these types of questions 1.Area of a square with length of side
2.Area of a circle with radius
3.Area of semicircle with radius of circle
4.Area of quarter circle with radius of circle
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

Write five sentences about Earth class 8 biology CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

In a school there are two sections of class X section class 8 maths CBSE

Explain land use pattern in India and why has the land class 8 social science CBSE




