Answer
453.3k+ views
Hint:
We will first find the area of the parallelogram using the formula for the area of a parallelogram. Then we will find the area of the rectangle using the formula for the area of a rectangle. We will compare both the areas to prove that both the areas are equal.
Formulas used: We will use the following formulas:
1) The area of a rectangle \[{A_r} = l \times b\], where \[l\] is the length and \[b\] is the base.
2) The area of a parallelogram, \[{A_p} = b \times h\] , where \[h\] is the height and \[b\] is the base.
Complete step by step solution:
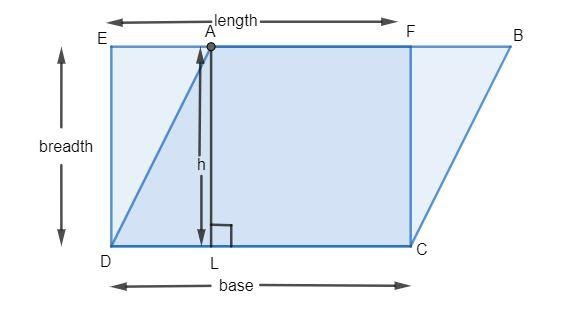
We will first prove the \[{\rm{ar}}\left( {ABCD} \right) = DC \times AL\].
(ii) We will find the area of parallelogram ABCD. We know that the area of a parallelogram is the product of the length of its height and base. So, we can write
\[ar\left( {ABCD} \right) = b \times h\]
The height of a parallelogram is the perpendicular distance between its 2 parallel opposite sides and the base of the parallelogram is its lowermost side. We can see from the figure that the height of the parallelogram is \[AL\] and the base is \[DC\]. We will substitute these values in the formula for the area of a parallelogram:
\[ \Rightarrow ar\left( {ABCD} \right) = AL \times DC\]
Hence, we have proved \[{\rm{ar}}\left( {ABCD} \right) = DC \times AL\].
Now, we will prove the \[{\rm{ar}}\left( {ABCD} \right) = {\rm{ar}}\left( {EFCD} \right)\].
We will find the area of the rectangle EFCD. We know that the area of a rectangle is its length times breadth.
\[ \Rightarrow ar\left( {EFCD} \right) = {\rm{length}} \times {\rm{breadth}}\]
Usually, the longer side of a rectangle is taken as its length and the shorter side is taken as its breadth. The breadth of a rectangle is always perpendicular to its length just like the height of a parallelogram. We can see from the figure that the length of the rectangle is \[EF\] and the breadth is \[ED\]. We will substitute these values in the formula for the area of a rectangle.
Therefore,
\[ \Rightarrow ar\left( {EFCD} \right) = EF \times ED\]
We know that the opposite sides of a rectangle are equal in length. So,
\[EF = DC\]
We can see from the figure that the breadth of the rectangle and the height of the parallelogram are equal. So, we can write
\[ED = AL\]
So, the area of rectangle EFCD can be rewritten as:
\[ \Rightarrow ar\left( {EFCD} \right) = DC \times AL\]
We know that the area of parallelogram ABCD is also \[AL \times DC\].
$\therefore ar\left( ABCD \right)=ar\left( EFCD \right)$
Note:
A parallelogram is a quadrilateral whose opposite sides are parallel and equal. A rectangle is a special kind of parallelogram with all its angles as right angles. The area of a rectangle is also the same as the area of a parallelogram as the base of the parallelogram is the rectangle’s length and the height of the parallelogram is the rectangle’s breadth.
We will first find the area of the parallelogram using the formula for the area of a parallelogram. Then we will find the area of the rectangle using the formula for the area of a rectangle. We will compare both the areas to prove that both the areas are equal.
Formulas used: We will use the following formulas:
1) The area of a rectangle \[{A_r} = l \times b\], where \[l\] is the length and \[b\] is the base.
2) The area of a parallelogram, \[{A_p} = b \times h\] , where \[h\] is the height and \[b\] is the base.
Complete step by step solution:
We will first prove the \[{\rm{ar}}\left( {ABCD} \right) = DC \times AL\].
(ii) We will find the area of parallelogram ABCD. We know that the area of a parallelogram is the product of the length of its height and base. So, we can write
\[ar\left( {ABCD} \right) = b \times h\]
The height of a parallelogram is the perpendicular distance between its 2 parallel opposite sides and the base of the parallelogram is its lowermost side. We can see from the figure that the height of the parallelogram is \[AL\] and the base is \[DC\]. We will substitute these values in the formula for the area of a parallelogram:
\[ \Rightarrow ar\left( {ABCD} \right) = AL \times DC\]
Hence, we have proved \[{\rm{ar}}\left( {ABCD} \right) = DC \times AL\].
Now, we will prove the \[{\rm{ar}}\left( {ABCD} \right) = {\rm{ar}}\left( {EFCD} \right)\].
We will find the area of the rectangle EFCD. We know that the area of a rectangle is its length times breadth.
\[ \Rightarrow ar\left( {EFCD} \right) = {\rm{length}} \times {\rm{breadth}}\]
Usually, the longer side of a rectangle is taken as its length and the shorter side is taken as its breadth. The breadth of a rectangle is always perpendicular to its length just like the height of a parallelogram. We can see from the figure that the length of the rectangle is \[EF\] and the breadth is \[ED\]. We will substitute these values in the formula for the area of a rectangle.
Therefore,
\[ \Rightarrow ar\left( {EFCD} \right) = EF \times ED\]
We know that the opposite sides of a rectangle are equal in length. So,
\[EF = DC\]
We can see from the figure that the breadth of the rectangle and the height of the parallelogram are equal. So, we can write
\[ED = AL\]
So, the area of rectangle EFCD can be rewritten as:
\[ \Rightarrow ar\left( {EFCD} \right) = DC \times AL\]
We know that the area of parallelogram ABCD is also \[AL \times DC\].
$\therefore ar\left( ABCD \right)=ar\left( EFCD \right)$
Note:
A parallelogram is a quadrilateral whose opposite sides are parallel and equal. A rectangle is a special kind of parallelogram with all its angles as right angles. The area of a rectangle is also the same as the area of a parallelogram as the base of the parallelogram is the rectangle’s length and the height of the parallelogram is the rectangle’s breadth.
Recently Updated Pages
What is the maximum resistance which can be made using class 10 physics CBSE

Arrange the following elements in the order of their class 10 chemistry CBSE

In the following figure the value of resistor to be class 10 physics CBSE

The magnetic induction at point P which is at a distance class 10 physics CBSE

According to Mendeleevs Periodic Law the elements were class 10 chemistry CBSE

Fill in the blanks with suitable prepositions Break class 10 english CBSE

Trending doubts
When was Karauli Praja Mandal established 11934 21936 class 10 social science CBSE

Name five important trees found in the tropical evergreen class 10 social studies CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Change the following sentences into negative and interrogative class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

Explain the Treaty of Vienna of 1815 class 10 social science CBSE