
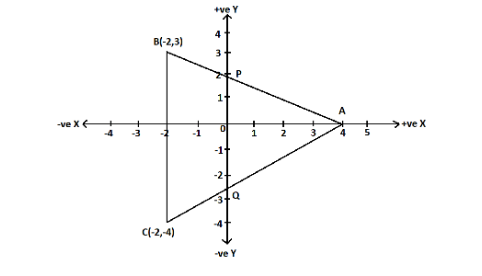
In the given figure ABC is a triangle and BC is parallel to the y-axis. AB and AC intersect the y-axis at P and Q respectively.
(i) Write the coordinates of A.
(ii) Find the length of AB and AC.
(iii) Find the ratio in which Q divides AC.
(iv) Find the equation of the line AC.

Answer
477.3k+ views
Hint: We can clearly see where the point A is, So note down its coordinates from the diagram. Find the length of AB and AC using distance between two points formula which is mentioned below. The ratio in which Q divides AC can also be calculated using the below mentioned formula. Equation of Ac is found by using two points form a line equation.
1. Distance between two points
2. If a point P divides a line in the ratio m:n, then its coordinates will be
3. Two points form of a line equation is
Complete step-by-step answer:
We are given that ABC is a triangle and BC is parallel to the y-axis.
(i) Coordinates of A is
So, the correct answer is “ {4,0}”.
(ii) Length of AB and AC
In AB,
Length of AB is
In AC,
Length of AC is
So, the correct answer is “
(iii) Ratio in which Q divides AC.
Here Ratio is m:n and
As we can see, Q is on the y-axis, so its x-coordinate will be zero.
Therefore, the coordinates of Q is
Corresponding coordinates are equal.
Therefore,
Therefore, Q divides AC in the ratio 2:1.
So, the correct answer is “2:1”.
(iv) Equation of line AC.
Here the two points are
Two points form of a line equation is
This gives,
The equation of line AC is
So, the correct answer is “
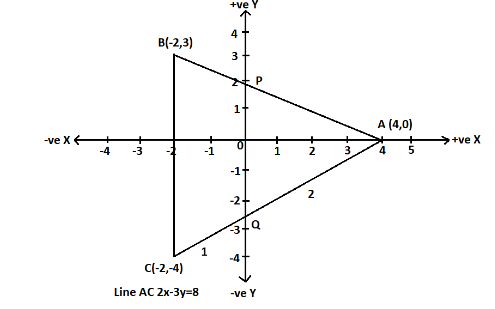
Note: Distance is a measurement, so it will always have units. If units are not mentioned in the question, you can just put the word “units” after the distance value. When two points are given, we can also find the line equation by finding the slope of the line and then using the slope-point form.
1. Distance between two points
2. If a point P divides a line in the ratio m:n, then its coordinates will be
3. Two points form of a line equation is
Complete step-by-step answer:
We are given that ABC is a triangle and BC is parallel to the y-axis.
(i) Coordinates of A is
So, the correct answer is “ {4,0}”.
(ii) Length of AB and AC
In AB,
Length of AB is
In AC,
Length of AC is
So, the correct answer is “
(iii) Ratio in which Q divides AC.
Here Ratio is m:n and
As we can see, Q is on the y-axis, so its x-coordinate will be zero.
Therefore, the coordinates of Q is
Corresponding coordinates are equal.
Therefore,
Therefore, Q divides AC in the ratio 2:1.
So, the correct answer is “2:1”.
(iv) Equation of line AC.
Here the two points are
Two points form of a line equation is
This gives,
The equation of line AC is
So, the correct answer is “

Note: Distance is a measurement, so it will always have units. If units are not mentioned in the question, you can just put the word “units” after the distance value. When two points are given, we can also find the line equation by finding the slope of the line and then using the slope-point form.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




