
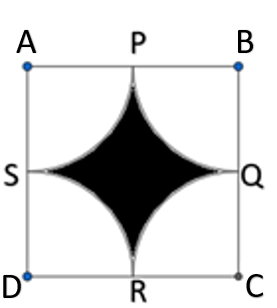
In the given figure ABCD is a square whose sides are of length 2cm. The mid points of side AB, BC, CD and DA are respectively P, Q, R and S. four arcs are drawn with A, B, C and D as centres and AP, BQ, RD and SA as radius. Find the area of the shaded portion.

Answer
495.3k+ views
Hint: In the figure, area of shaded portion is given by Area of square – Area of all 4 quarter circle. We know the formula for area of square is
Complete step by step answer:
Now first consider the diagram given.
In this figure ABCD is a square with each side as 2cm.
Hence we have AB = BC = CD = DA = 2cm ……………………….. (1)
Now we know that Area is square is given by
Hence Area of square ABCD is
Now consider 4 quarter circles.
Now we have that P, Q, R and S are mid points of each side respectively. Hence from equation (1) we can say AP = BQ = RD = SA =
Now these are nothing but radius of respective quarter circles.
Now area of quarter circle is given by
Now since radius of each quarter circle is equal their Area will also be equal.
Hence we get radius of each quarter circle =
Now we can see that the area of shaded portion is nothing but Area of square – Area of 4 quarter circles.
Hence, from equation (2) and (3) we get.
Area of shaded region
Taking – 1 common we get
Hence area of shaded portion is
So, the correct answer is “Option b”.
Note:
We know that 4 quarter circles of same radius is equal to circle. Hence we can directly solve this questions by replacing area of 4 quarter circles of radius 1cm by area of circle with radius 1cm and hence the formula for shaded region would be area of square – area of circle with radius 1cm.
Complete step by step answer:
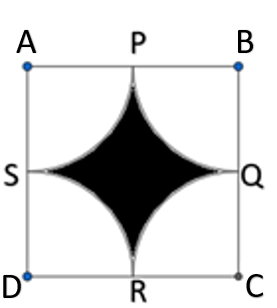
Now first consider the diagram given.

In this figure ABCD is a square with each side as 2cm.
Hence we have AB = BC = CD = DA = 2cm ……………………….. (1)
Now we know that Area is square is given by
Hence Area of square ABCD is
Now consider 4 quarter circles.
Now we have that P, Q, R and S are mid points of each side respectively. Hence from equation (1) we can say AP = BQ = RD = SA =
Now these are nothing but radius of respective quarter circles.
Now area of quarter circle is given by
Now since radius of each quarter circle is equal their Area will also be equal.
Hence we get radius of each quarter circle =
Now we can see that the area of shaded portion is nothing but Area of square – Area of 4 quarter circles.
Hence, from equation (2) and (3) we get.
Area of shaded region
Taking – 1 common we get
Hence area of shaded portion is
So, the correct answer is “Option b”.
Note:
We know that 4 quarter circles of same radius is equal to circle. Hence we can directly solve this questions by replacing area of 4 quarter circles of radius 1cm by area of circle with radius 1cm and hence the formula for shaded region would be area of square – area of circle with radius 1cm.
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




