Question

In the given figure $AB\parallel PQ$ . If PQ=1.5cm, QC=2cm and BQ=8cm, then the measure of AB is:

a.10cm
b.7.5cm
c.9.5cm
d.3.5cm

Answer 1

Hint: We can use the concept of similarity of triangles and then use the ratio of the corresponding sides in order to obtain the required answer.

Complete step by step answer:
Let the figure below represent the given situation.

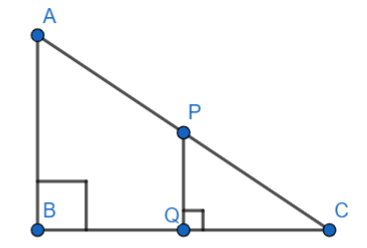
It is given that the lengths of PQ, QC and QB are 1.5cm, 2cm and 8cm respectively, i.e.
PQ=1.5cm
QC=2cm
QB=8cm
We are going to find the length of side AB using the properties of similar triangles.
Two angles are said to be similar if their angles are the same. As the sum of angles of a triangle should be ${180}^{\circ }$, two triangles are similar if any two angles are similar. This is known as the A-A similarity criterion. ……………………… (1.1)
In case of similar triangles, the ratio of sides between the same set of angles is fixed. ……………………. (1.2)
Now,
In $\mathrm{\Delta }ABC$ and $\mathrm{\Delta }PQC$,
$\mathrm{\angle }ABC=\mathrm{\angle }PQC\text{ (Each }{90}^{\circ }\text{)}$
$\mathrm{\angle }ACB=\mathrm{\angle }PCQ\text{ (Common angle C)}$
So, by the A-A similarity criterion stated in (1.1), $$\mathrm{\Delta }ABC\cong \mathrm{\Delta }PQC$$ .
Now, we can use property (1.2) of similar triangles to the triangles to obtain:
${\displaystyle \frac{AB}{PQ}}={\displaystyle \frac{BC}{QC}}={\displaystyle \frac{AC}{PC}}$
$\Rightarrow {\displaystyle \frac{AB}{PQ}}={\displaystyle \frac{BQ+QC}{QC}}$
$\Rightarrow {\displaystyle \frac{AB}{1.5}}={\displaystyle \frac{8+2}{2}}$
$\Rightarrow {\displaystyle \frac{AB}{1.5}}=5$
$\Rightarrow AB=5\times 1.5$
$\Rightarrow AB=7.5cm$
Thus, by using the concept of similarity of triangles we find that the length of AB is 7.5cm.
This matches the answer given in option (b). Hence, the required correct option is option (b)7.5cm.

Note: We should be careful that in case of similar triangles, only the ratio of the sides between two angles will be equal and do not depend on the magnitudes of their length. Therefore, we should be careful about taking the ratio of the sides such that they enclose the same angles.