
In the given figure, AOC is a straight line. If angle AOB =
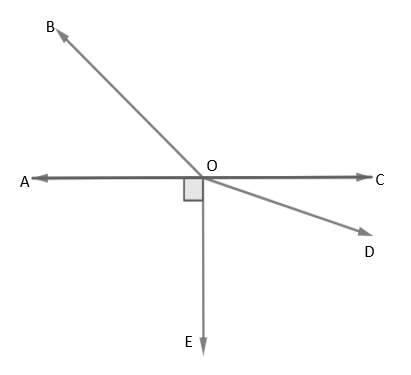
(i) angle BOC
(ii) angle EOD
(iii) obtuse angle BOD
(iv) reflex angle BOD
(v) reflex angle COE

Answer
499.2k+ views
Hint:We solve this question by first drawing the figure and representing the given angles. Then we use the fact that the angle made by a straight line on one side is
Complete step by step answer:
We are given that in the given figure, angle AOB=
Then let us now show those values in the figure. So, it looks like,
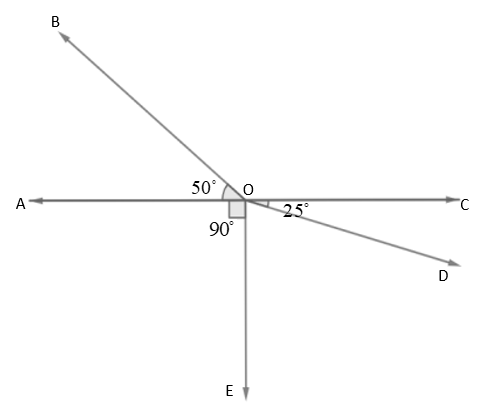
(i) angle BOC.
We are given that AC is a straight line.
Let us consider the fact that the angle made by a straight line on one side is
Using this we can say that angle made by line AC on the side with point B is
So, we get,
Now let us substitute the value of angle AOB in it. Then we get,
Hence answer is
(ii) angle EOD.
The form above we can say the angle made by AC on the other side is also
So, we get that
Now let us substitute the value of angle AOE in it. Then we get,
We can write angle EOC as,
Substituting the value of angle DOC, we get,
Hence answer is
(iii) obtuse angle BOD.
From the figure above, we can say that,
Now let us substitute the value of angle COD which is given and angle BOC from above equation (1).
Then we get,
Hence answer is
(iv) reflex angle BOD
First, let us consider the concept of reflex angle.
A reflex angle is the larger angle made between the points.
Here from the above subpart (iii) we know that angle BOD is
As we need to find the other possible angle, let us subtract it from the total angle around the line, that is
Hence answer is
(v) reflex angle COE
First, let us consider the concept of reflex angle.
A reflex angle is the larger angle made between the points.
Here from the above subpart (ii) we know that angle COE is
As we need to find the other possible angle, let us subtract it from the total angle around the line, that is
Hence answer is
Note:
The common mistake one makes while solving this problem is one might subtract the smaller angle from
Complete step by step answer:
We are given that in the given figure, angle AOB=
Then let us now show those values in the figure. So, it looks like,

(i) angle BOC.
We are given that AC is a straight line.
Let us consider the fact that the angle made by a straight line on one side is
Using this we can say that angle made by line AC on the side with point B is
So, we get,
Now let us substitute the value of angle AOB in it. Then we get,
Hence answer is
(ii) angle EOD.
The form above we can say the angle made by AC on the other side is also
So, we get that
Now let us substitute the value of angle AOE in it. Then we get,
We can write angle EOC as,
Substituting the value of angle DOC, we get,
Hence answer is
(iii) obtuse angle BOD.
From the figure above, we can say that,
Now let us substitute the value of angle COD which is given and angle BOC from above equation (1).
Then we get,
Hence answer is
(iv) reflex angle BOD
First, let us consider the concept of reflex angle.
A reflex angle is the larger angle made between the points.
Here from the above subpart (iii) we know that angle BOD is
As we need to find the other possible angle, let us subtract it from the total angle around the line, that is
Hence answer is
(v) reflex angle COE
First, let us consider the concept of reflex angle.
A reflex angle is the larger angle made between the points.
Here from the above subpart (ii) we know that angle COE is
As we need to find the other possible angle, let us subtract it from the total angle around the line, that is
Hence answer is
Note:
The common mistake one makes while solving this problem is one might subtract the smaller angle from
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Discuss the main reasons for poverty in India




