
In the given figure mass of string AB is 2 kg. Find tensions at A, B and C, where C is the midpoint of string.
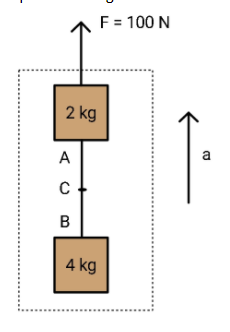

Answer
495k+ views
1 likes
Hint: Consider drawing free body diagrams for different segments in question. Start by considering the entire system to be an isolated block and determine the acceleration for the entire system first.
Complete answer:
We first take the sum of all the masses involved i.e., 2 kg of the string, total 6 kg for the two blocks should give us, total mass of 8 kg. Now this mass happens to be moving by an acceleration determined by considering the free body equation:

as weight W of the entire system acts downwards and F is pulling the system upwards. For simplification, we determine W to be 80 N for the system as we consider g to be 10 ms
(we are already given F = 100 N).
This is the acceleration with which all the components inside the system are moving.
Now we break the problem into three segments and construct the following three cases.
1. To determine the tension at point A, consider the following free body diagram consisting of the entire chain length AB and the mass underneath it of 4 kg.
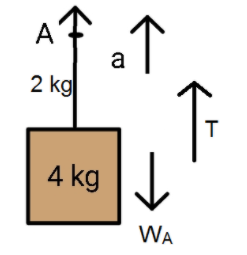
Looking at the diagram, we may write:
where
The mass concerned here, happens to be 2 kg of the string and 4 kg of the block so,
Keeping the values in the free body equation we had, we get:
This means that tension at point A is 75 N.
2. Now, point C happens to be at the midpoint of the string, so we take only half the mass of the string i.e., 1 kg and we have the 4 kg block too, so,
We have the free body diagram same as before, but the equation now becomes:
Keeping the values we get:
This means that attention at point C is 62.5 N.
3. For point B, the free body diagram now consists of only the block of 4 kg.
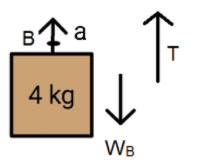
The equation for this is:
where
Therefore the tension in the string at point B is 50 N.
Note:
In writing the free body equation we considered the upward force direction to be positive and downward force direction to be negative. We write the resultant force as the sum of forces acting on the body.
Complete answer:
We first take the sum of all the masses involved i.e., 2 kg of the string, total 6 kg for the two blocks should give us, total mass of 8 kg. Now this mass happens to be moving by an acceleration determined by considering the free body equation:

as weight W of the entire system acts downwards and F is pulling the system upwards. For simplification, we determine W to be 80 N for the system as we consider g to be 10 ms
(we are already given F = 100 N).
This is the acceleration with which all the components inside the system are moving.
Now we break the problem into three segments and construct the following three cases.
1. To determine the tension at point A, consider the following free body diagram consisting of the entire chain length AB and the mass underneath it of 4 kg.

Looking at the diagram, we may write:
where
The mass concerned here, happens to be 2 kg of the string and 4 kg of the block so,
Keeping the values in the free body equation we had, we get:
This means that tension at point A is 75 N.
2. Now, point C happens to be at the midpoint of the string, so we take only half the mass of the string i.e., 1 kg and we have the 4 kg block too, so,
We have the free body diagram same as before, but the equation now becomes:
Keeping the values we get:
This means that attention at point C is 62.5 N.
3. For point B, the free body diagram now consists of only the block of 4 kg.

The equation for this is:
where
Therefore the tension in the string at point B is 50 N.
Note:
In writing the free body equation we considered the upward force direction to be positive and downward force direction to be negative. We write the resultant force as the sum of forces acting on the body.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




