
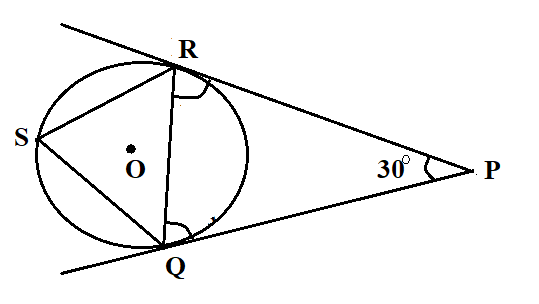
In the given figure,

Answer
494.4k+ views
Hint: We use the theorem that tangents from the common point are equal and then calculate the values of
Complete step-by-step answer:
We are given the value of
We have to find the value of
Here, we can see that tangents
It is known that angles opposite to equal sides are equal in a triangle
Therefore,
And sum of all the angles of a triangle is
For triangle
On substituting the value
Therefore, we have
Now,
Then,
Also, the angle between the chord and tangent is equal to the angle in the alternate segment.
Therefore,
Hence, in triangle,
Therefore,
And the sum of all the angles of a triangle is
Hence, the value of
Note: Many students make mistakes by assuming that
Complete step-by-step answer:
We are given the value of
We have to find the value of
Here, we can see that tangents
It is known that angles opposite to equal sides are equal in a triangle
Therefore,
And sum of all the angles of a triangle is
For triangle
On substituting the value
Therefore, we have
Now,
Then,
Also, the angle between the chord and tangent is equal to the angle in the alternate segment.
Therefore,
Hence, in triangle,
Therefore,
And the sum of all the angles of a triangle is
Hence, the value of
Note: Many students make mistakes by assuming that
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

Write a pair of integers whose sum gives i Zero ii class 7 maths CBSE

List of coprime numbers from 1 to 100 class 7 maths CBSE





