Question

In triangle ABC, AB = AC = 15 cm and BC = 18 cm.
Find \[\cos \angle ABC\] is \[\dfrac{3}{m},\text{ }m\] is
     \[\]

Answer 1

Hint: Draw\[\vartriangle ABC\] with the given length of sides. It is said that the AB = AC = 15 cm, as 2 sides are equal it is an isosceles triangle. Draw \[AD\bot BC\] .
Consider\[\vartriangle ABD\]. \[\angle ABD=\angle ABC\] and D is a point on line BC. Thus using basic trigonometric functions. Find \[\cos \angle ABC\] and compare its value with \[\cos \angle ABC=\dfrac{3}{m}\] to get the value of \[m\].
Complete step-by-step answer:
Given to us the length of sides of a triangle ABC. Let us first draw a \[\vartriangle ABC\] . Mark the sides as
\[AB=15cm,AC=15cm\text{ }and\text{ BC=18cm}\text{.}\]

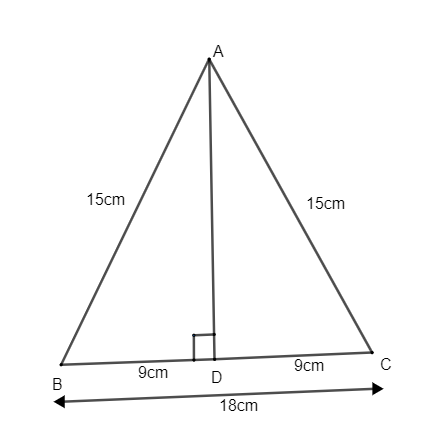
From this we can say that the 2 lengths of \[\vartriangle ABC\] are the same, which means that \[\vartriangle ABC\] is an isosceles triangle.
Now, let us draw AD perpendicular from A to line BC, such that \[AD\bot BC\]. Now AD bisects BC such that it divides BC into two equal halves.
\[\begin{align}
  & BC=BD+DC \\
 & \\
 & \therefore BD=DC=\dfrac{18}{2}=9cm \\
 & \\
 & As\text{ }AD\bot BC,\text{ }\angle D={{90}^{\circ }} \\
\end{align}\]
Now let us consider the right \[\vartriangle ADB\] from the figure drawn.
The base of \[\vartriangle ADB\] is BD = 9cm.
Hypotenuse of the \[\vartriangle ADB\] is AB = 15 cm.
\[\angle ABD=\angle ABC\] as D is a point on the line BC.
\[\therefore \cos \angle ABD=\cos \angle ABC=\dfrac{adjacent\text{ }sides}{hypotenuse}=\dfrac{9}{15}\]
\[\therefore \cos \angle ABD=\dfrac{3}{5}\], by simplification \[\to (1)\]
Now we have been given \[\cos \angle ABC=\dfrac{3}{m}\], we need to find the value of \[m\].
By comparing both (1) and \[\cos \angle ABC=\dfrac{3}{m}\]
We got the value of \[m=5\].
Hence we \[\cos \angle ABC=\dfrac{3}{5}\].
Thus we got the required value.

Note: From the given length, AB = AC = 15 cm, you should be able to identify that it is an isosceles triangle. Draw \[AD\bot BC\], which is an important step here, without doing this you won’t get right \[\vartriangle ADB\] to find the value of \[\angle ABD\].