Question

In $\vartriangle ABC$, \[CD \bot AB\], \[CA = 2AD\] and \[BD = 3AD\]. Prove that \[\angle BCA = 90^\circ \].

Answer 1

Hint: Here, we will prove \[\angle BCA = 90^\circ \] by using Pythagoras theorem. First we will draw the diagram based on the given information. We will apply Pythagoras theorem in all the right angle triangles to find unknown sides. Pythagoras theorem is applied only to right angled triangles and not other triangles. It states that the square of hypotenuse is equal to the sum of squares of the other two sides. Hypotenuse is the longest side.

Formula Used:
Pythagoras Theorem, \[{h^2} = {a^2} + {b^2}\] , where, \[h\]is the hypotenuse and \[a\] and \[b\] are the length of the base and the perpendicular sides respectively.

Complete step-by-step answer:
We will draw a $\vartriangle ABC$ and then draw a perpendicular from the vertex \[C\] to \[AB\] such that \[CD \bot AB\].
Also, let the length of the side \[AD = x\]
Hence, according to the question,
Since, \[CA = 2AD\]
Therefore, \[CA = 2AD = 2x\]
Also, \[BD = 3AD = 3x\]

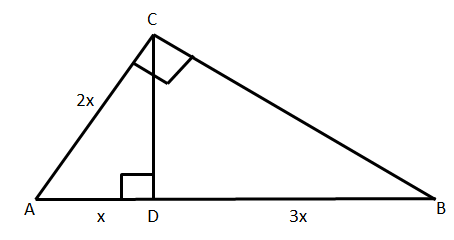
Now, in $\vartriangle ADC$ which is right angled at \[\angle D\] (Because \[CD \bot AB\]),
We will apply Pythagoras Theorem, i.e.
 \[{h^2} = {a^2} + {b^2}\] , where, \[h\] is the hypotenuse and \[a\] and \[b\] are the length of the base and the perpendicular sides respectively.
Hence, by applying Pythagoras theorem in $\vartriangle ADC$,
 \[{\left( {AC} \right)^2} = {\left( {AD} \right)^2} + {\left( {CD} \right)^2}\]
 \[ \Rightarrow {\left( {2x} \right)^2} = {\left( x \right)^2} + {\left( {CD} \right)^2}\]
Hence, solving further,
 \[ \Rightarrow {\left( {CD} \right)^2} = 4{x^2} - {x^2} = 3{x^2}\]
Taking square root on both sides,
 \[ \Rightarrow CD = \sqrt 3 x\]
Hence, the length of \[CD = \sqrt 3 x\]
Also, after taking the square root, we have not considered the negative value because the length of the side of a triangle can never be negative.
Now, similarly, we will apply the Pythagoras Theorem in $\vartriangle CDB$ which is right angled at \[\angle D\] (Because \[CD \bot AB\]),
 \[{\left( {BC} \right)^2} = {\left( {BD} \right)^2} + {\left( {CD} \right)^2}\]
 \[ \Rightarrow {\left( {BC} \right)^2} = {\left( {3x} \right)^2} + {\left( {\sqrt 3 x} \right)^2}\]
Hence, solving further,
 \[ \Rightarrow {\left( {BC} \right)^2} = 9{x^2} + 3{x^2} = 12{x^2}\]
Taking square root on both sides,
 \[ \Rightarrow BC = 2\sqrt 3 x\]
Hence, the length of \[BC = 2\sqrt 3 x\]
Again, after taking the square root, we have not considered the negative value because the length of the side of a triangle can never be negative.
Now, finally in order to prove that \[\angle BCA = 90^\circ \],
We will try to prove that $\vartriangle BCA$ is a right angled triangle right angled at \[\angle C\].
Hence, applying Pythagoras Theorem in $\vartriangle BCA$,
 \[{\left( {AB} \right)^2} = {\left( {AC} \right)^2} + {\left( {BC} \right)^2}\]
Now, the length of side \[AB = AD + BD = x + 3x = 4x\]
And, it is given that \[AC = 2x\]
Also, we have proved that \[BC = 2\sqrt 3 x\]
 \[ \Rightarrow {\left( {4x} \right)^2} = {\left( {2x} \right)^2} + {\left( {2\sqrt 3 x} \right)^2}\]
 \[ \Rightarrow 16{x^2} = 4{x^2} + 12{x^2}\]
Hence, solving further,
 \[ \Rightarrow 16{x^2} = 16{x^2}\]
Clearly, LHS \[ = \]RHS
Therefore, Pythagoras theorem holds true in this triangle.
Hence, $\vartriangle BCA$ is a right angled triangle right angled at \[\angle C\].
Therefore, it is proved that \[\angle BCA = 90^\circ \]
Hence, proved

Note: An alternate way to solve this question is:
We will use trigonometric identities in a right angled triangle.
As we know, in a right angled triangle \[\cos \theta = \dfrac{B}{H}\], where \[B\]is the base and \[H\] is the hypotenuse.
Therefore, in $\vartriangle ADC$
 \[\cos \theta = \dfrac{B}{H} = \dfrac{{AD}}{{AC}} = \dfrac{x}{{2x}} = \dfrac{1}{2}\]
Now, using trigonometric tables, we know that \[\cos 60^\circ = \dfrac{1}{2}\]
 \[ \Rightarrow \cos \theta = \cos 60^\circ \]
Therefore, \[\angle CAD = \theta = 60^\circ \]

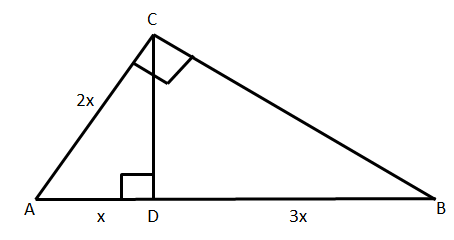
Now, let us assume that \[\angle BCA \ne 90^\circ \]
Hence, in $\vartriangle BCA$,
 \[\cos \theta = \dfrac{B}{H} = \dfrac{{AC}}{{AB}} = \dfrac{{2x}}{{4x}} = \dfrac{1}{2}\]
Now, using trigonometric tables, we know that \[\cos 60^\circ = \dfrac{1}{2}\]
 \[ \Rightarrow \cos \theta = \cos 60^\circ \]
Therefore, \[\angle CAD = \theta = 60^\circ \]
But, we have already proved that \[\angle CAD = \theta = 60^\circ \]
Hence, this is a contradictory situation.
Therefore, our assumption is not correct and \[\angle BCA = 90^\circ \]
Hence, proved