
In YDSE the intensity of the central bright fringe is
A)
B)
C)
D)
Answer
495.3k+ views
Hint-YDSE is Young’s Double Slit Experiment. YDSE is performed with a monochromatic light source. Coherent source of light: Two sources of light are said to be a coherent source if the phase difference between them is constant and the same frequency.
Complete step by step answer:
Given details:
The intensity of central bright fringe,
Path difference,
Formula used:
where
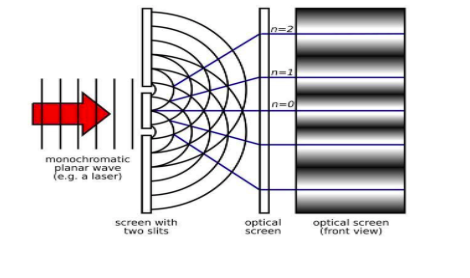
Monochromatic source of light:
The light having the same wavelength is called monochromatic light.
Interference of light:
When two monochromatic light waves are superimposed on each other, the intensity in the region of superposition gets redistributed, becoming maximum at some points and minimum at others.
In an interference pattern, the intensities at the points of maxima and minima are directly proportional to the square of the amplitude of the waves. If there is no interference between the light waves from the two sources, then the intensity at every point will be the same and there will be no formation of fringes.
Therefore, we can calculate the value of phase difference.
The phase difference is given by
Now, we will calculate the intensity at a path difference (
The correct option is (B).
Note:1. The intensity of light produced by Young’s Double Slit Experiment is calculated as
2. Bright fringes or constructive interference are produced when the value of
3. Dark fringes or destructive interference are produced when the value of
4. According to Huygens’ principle a cylindrical wavefront emerges from a point source, in Young’s Double Slit Experiment point source is used so the wavefronts are cylindrical wavefront.
Complete step by step answer:
Given details:
The intensity of central bright fringe,
Path difference,
Formula used:
where

Monochromatic source of light:
The light having the same wavelength is called monochromatic light.
Interference of light:
When two monochromatic light waves are superimposed on each other, the intensity in the region of superposition gets redistributed, becoming maximum at some points and minimum at others.
In an interference pattern, the intensities at the points of maxima and minima are directly proportional to the square of the amplitude of the waves. If there is no interference between the light waves from the two sources, then the intensity at every point will be the same and there will be no formation of fringes.
Therefore, we can calculate the value of phase difference.
The phase difference is given by
Now, we will calculate the intensity at a path difference (
The correct option is (B).
Note:1. The intensity of light produced by Young’s Double Slit Experiment is calculated as
2. Bright fringes or constructive interference are produced when the value of
3. Dark fringes or destructive interference are produced when the value of
4. According to Huygens’ principle a cylindrical wavefront emerges from a point source, in Young’s Double Slit Experiment point source is used so the wavefronts are cylindrical wavefront.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




