Question

Where is the incenter of the triangle \[\Delta ABC\]?

(a) A
(b) B
(c) I
(d) C

Answer 1

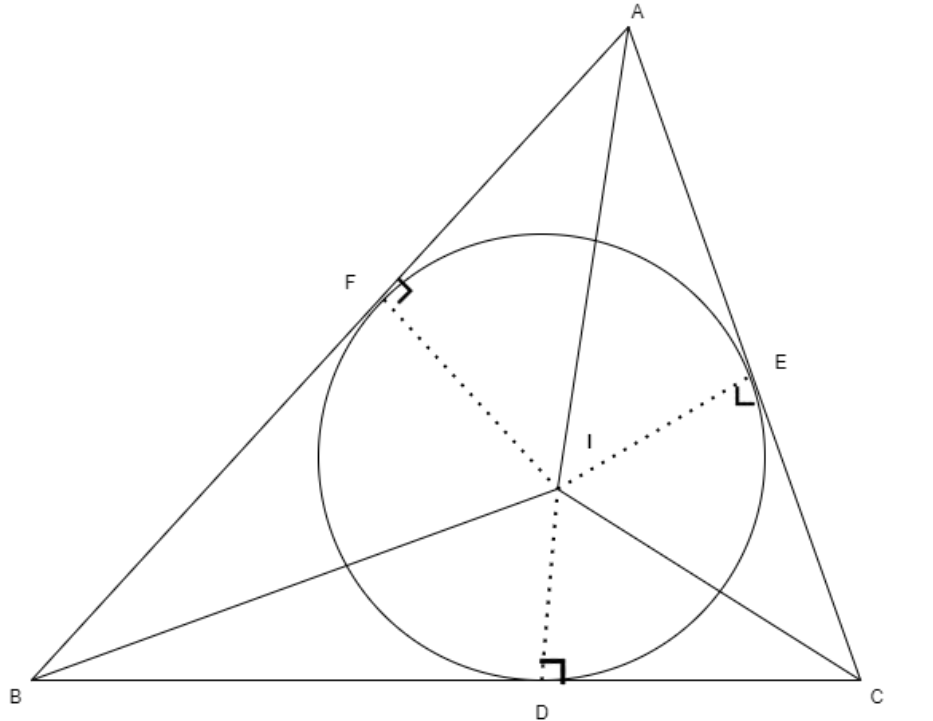
Hint: We need to find the incenter using definition and its locus. The incenter is defined as the point of intersection of three angular bisectors of the triangle. In other terms, it can be defined as the locus of points which is equidistant from the two sides taken at the time and passes through one vertex of the same sides. In the figure, if BI is the angular bisector of \[\angle ABC\] then \[ID=IF\]. By considering this we need to check which is the incenter of the given triangle.

Complete step-by-step solution
Let us assume that ‘I’ is the incenter of the triangle and we try to prove that BI, AI, CI are angular bisectors.
In the figure, we know that ‘I’ is the center of the circle, and ‘ID’, IE’, ‘IF’ are radii of the circle.
We know that the radii of the circle are always equal we can write,
\[ID=IE=IF\]
Let us consider \[ID=IF\], this means that ‘I’ is equidistant from both the sides ‘AB’ and ‘BC’.
Now let us consider\[IE=IF\], this means that ‘I’ is equidistant from both the sides ‘AB’, ‘CA’.
Now let us consider \[ID=IF\], this means that ‘I’ is equidistant from both the sides ‘BC’, ‘CA’.
Here, we know that if ‘I’ is the incenter of the triangle which lies on the angular bisector it needs to be equidistant from all the sides.
Since ‘I’ is equidistant from all the sides as shown above we can say that that lines ‘AI’, ‘BI’, and ‘CI’ are the angular bisectors of the respective angles.
We proved that ‘I’ is the incenter of the \[\Delta ABC\].

Note:We can solve this problem in the reverse order also. In this case, we consider that the lines ‘AI’, ‘BI’, and ‘CI’ are the angular bisectors of the respective angles and try to prove that \[ID=IE=IF\]. These solution steps include the exact opposite lines we wrote above. This is the second method of solving the question.