
What is the internal angle of a regular 17-sided polygon?
Answer
432.3k+ views
Hint: To find the internal angle of a regular 17-sided polygon, we will use the formula for the sum of interior angles of a polygon which is given by
Complete step by step solution:
We have to find the internal angle of a regular 17-sided polygon. We know that an interior angle of a polygon is an angle formed inside the two adjacent sides of a polygon. Or we can say that they are the angle measures at the interior part of a polygon.
We know that the sum of interior angles of a polygon is given by the formula
where, n is the number of sides of the polygon.
We are given a 17 sided polygon. Therefore,
We have subtract 2 from 17.
We have to multiply 180 and 15.
Therefore, the sum of all the angles in a 17 sided polygon is
Therefore, the measure of 1 interior angle can be obtained by dividing the sum of all the interior angles by the number of sides.
Let us substitute the values in the above formula.
The figure for a regular 17 sided-polygon called a heptadecagon is shown below.
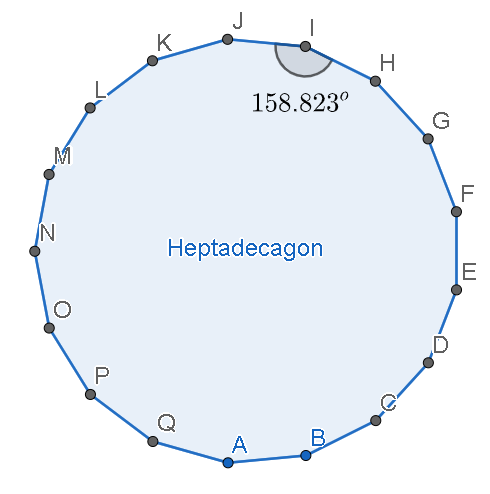
Therefore, the internal angle of a regular 17-sided polygon is
Note: Students must note the formula for the sum of the interior angles of a polygon remains the same for a regular and an irregular polygon but the individual angles vary in the latter. The sum of interior angles of a polygon is always a constant value. A polygon that does not have all sides equal and all angles equal. Is called irregular polygon in which a regular polygon will have all equal sides and equal angles. Students must never forget to put the symbol of degree after finding the measure of the angles.
Complete step by step solution:
We have to find the internal angle of a regular 17-sided polygon. We know that an interior angle of a polygon is an angle formed inside the two adjacent sides of a polygon. Or we can say that they are the angle measures at the interior part of a polygon.
We know that the sum of interior angles of a polygon is given by the formula
where, n is the number of sides of the polygon.
We are given a 17 sided polygon. Therefore,
We have subtract 2 from 17.
We have to multiply 180 and 15.
Therefore, the sum of all the angles in a 17 sided polygon is
Therefore, the measure of 1 interior angle can be obtained by dividing the sum of all the interior angles by the number of sides.
Let us substitute the values in the above formula.
The figure for a regular 17 sided-polygon called a heptadecagon is shown below.

Therefore, the internal angle of a regular 17-sided polygon is
Note: Students must note the formula for the sum of the interior angles of a polygon remains the same for a regular and an irregular polygon but the individual angles vary in the latter. The sum of interior angles of a polygon is always a constant value. A polygon that does not have all sides equal and all angles equal. Is called irregular polygon in which a regular polygon will have all equal sides and equal angles. Students must never forget to put the symbol of degree after finding the measure of the angles.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Discuss the main reasons for poverty in India




