
Is an isosceles triangle always, sometimes or never a right triangle?
Answer
403.2k+ views
Hint: A right triangle is a triangle where the measure of one angle is equal to
Complete step-by-step answer:
Let us consider that an isosceles triangle is always a right triangle.
So, for a right triangle an angle needs to be equal to
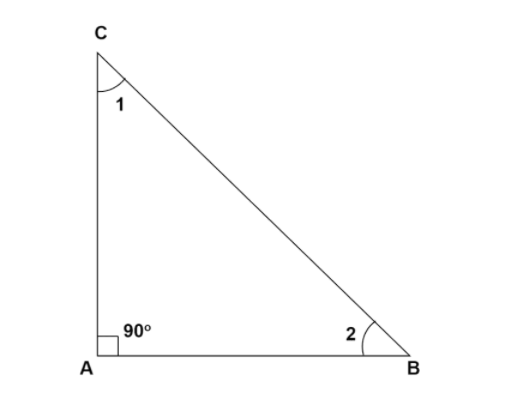
For a triangle to be an isosceles triangle, two angles need to be equal.
Let
Using Angle Sum Property, i.e. Sum of all the angles in a triangle
Using (1), we have
Solving the left hand side, we have
Reshuffling the terms, we get
Now, Dividing the whole equation by
Hence, we have,
Hence, an isosceles triangle is a right triangle if the measure of each of two angles other than the right angle is equal to
But this is not always true that an isosceles triangle is a right triangle.
Let us draw a diagram
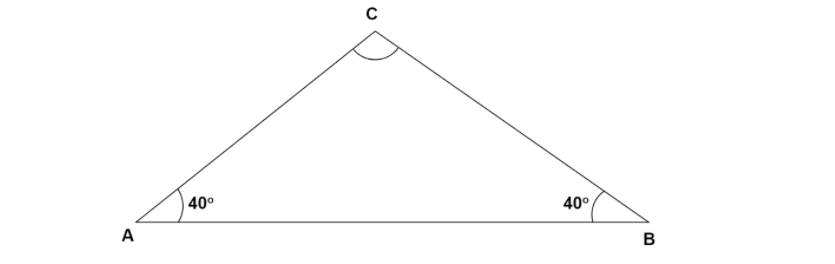
Let us consider a case where
Substituting
Solving the Left Hand side, we get
Reshuffling the terms, we get
Hence, we get
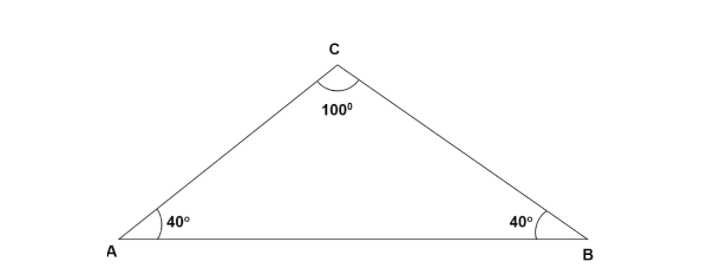
Now, we see that this triangle is an isosceles triangle but not a right triangle.
Hence, we can conclude that it is not always true that an isosceles triangle is a right triangle but An isosceles triangle is a right triangle if measure of one angle is equal to
Therefore, we can say that an isosceles triangle can be a right triangle sometimes.
So, the correct answer is “sometimes”.
Note: We need to consider every case to answer this question. We need to consider the conditions for both the triangles. None of the conditions can be relaxed. We can also consider the case where measure of each of two angles is
Complete step-by-step answer:
Let us consider that an isosceles triangle is always a right triangle.
So, for a right triangle an angle needs to be equal to

For a triangle to be an isosceles triangle, two angles need to be equal.
Let
Using Angle Sum Property, i.e. Sum of all the angles in a triangle
Using (1), we have
Solving the left hand side, we have
Reshuffling the terms, we get
Now, Dividing the whole equation by
Hence, we have,
Hence, an isosceles triangle is a right triangle if the measure of each of two angles other than the right angle is equal to
But this is not always true that an isosceles triangle is a right triangle.
Let us draw a diagram

Let us consider a case where
Substituting
Solving the Left Hand side, we get
Reshuffling the terms, we get
Hence, we get

Now, we see that this triangle is an isosceles triangle but not a right triangle.
Hence, we can conclude that it is not always true that an isosceles triangle is a right triangle but An isosceles triangle is a right triangle if measure of one angle is equal to
Therefore, we can say that an isosceles triangle can be a right triangle sometimes.
So, the correct answer is “sometimes”.
Note: We need to consider every case to answer this question. We need to consider the conditions for both the triangles. None of the conditions can be relaxed. We can also consider the case where measure of each of two angles is
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

List some examples of Rabi and Kharif crops class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

How many ten lakhs are in one crore-class-8-maths-CBSE




