
Let a focal chord of the parabola
A. -8
B. -16
C. 16
D. None of these
Answer
495.3k+ views
Hint: We here need to find
Complete step-by-step solution
Now, we have been given the two points the focal chord of the parabola
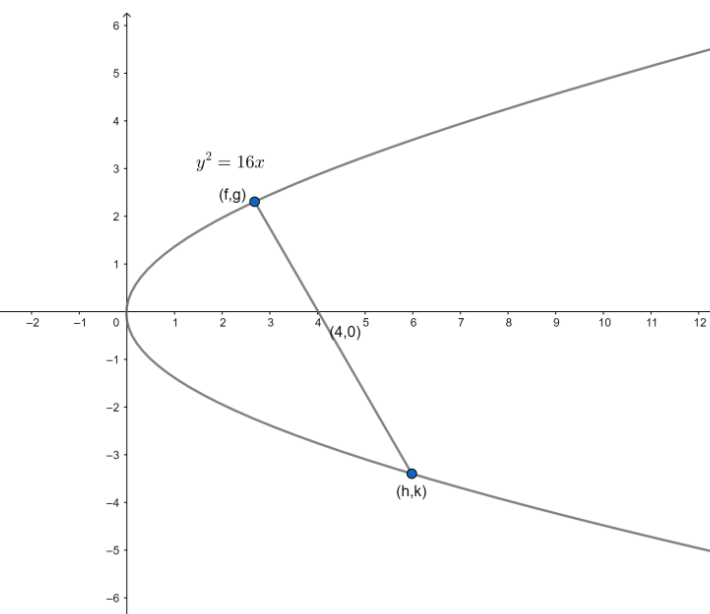
The parabola
Thus, for the given parabola,
Now, any point on a parabola
As mentioned above, here
Thus, we can take the given points
Thus, we get:
So we can get
Thus, we get:
Now, we know that if the focal chord of a parabola of the type
Thus, by putting this value, we can find the value of
Now, putting this value in equation (i) we get:
Thus option (C) is the correct option.
Note: We have obtained the result
In the given figure, we have a parabola
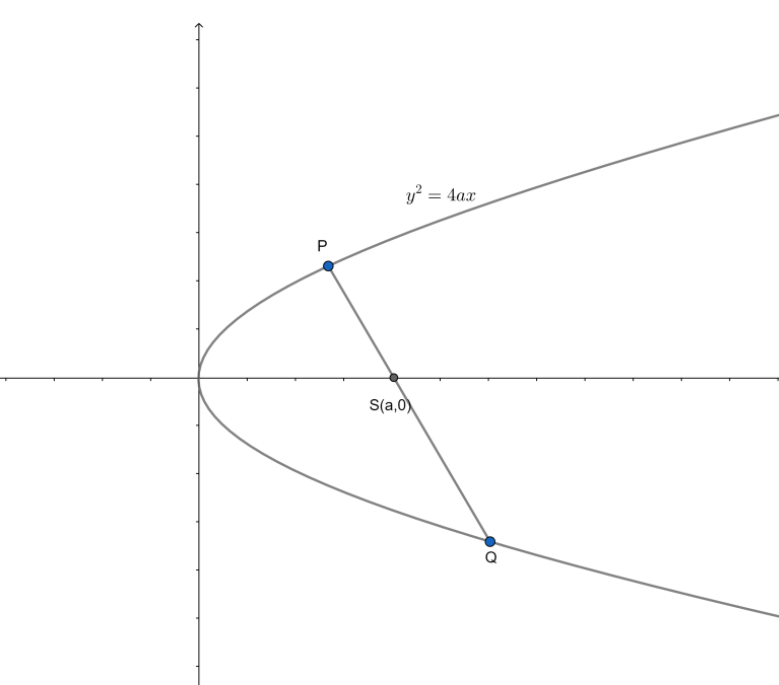
Let the point P be
Hence, the equation through point P and Q will be:
Now, this line passes through the focus, i.e. S (a,0)
Putting this point in the equation of the chord we get:
Complete step-by-step solution
Now, we have been given the two points the focal chord of the parabola

The parabola
Thus, for the given parabola,
Now, any point on a parabola
As mentioned above, here
Thus, we can take the given points
Thus, we get:
So we can get
Thus, we get:
Now, we know that if the focal chord of a parabola of the type
Thus, by putting this value, we can find the value of
Now, putting this value in equation (i) we get:
Thus option (C) is the correct option.
Note: We have obtained the result
In the given figure, we have a parabola

Let the point P be
Hence, the equation through point P and Q will be:
Now, this line passes through the focus, i.e. S (a,0)
Putting this point in the equation of the chord we get:
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




