
Let ABC be a triangle whose circumcentre is at P. If the position vectors of A, B, C and P are
(a)
(b)
(c)
(d)
Answer
481.5k+ views
Hint: We start solving the problem by recalling the fact that the centroid of the triangle with position vectors of vertices
Complete step by step answer:
According to the problem, we are given that ABC is a triangle whose circumcentre is at P and the position vectors of A, B, C and P are
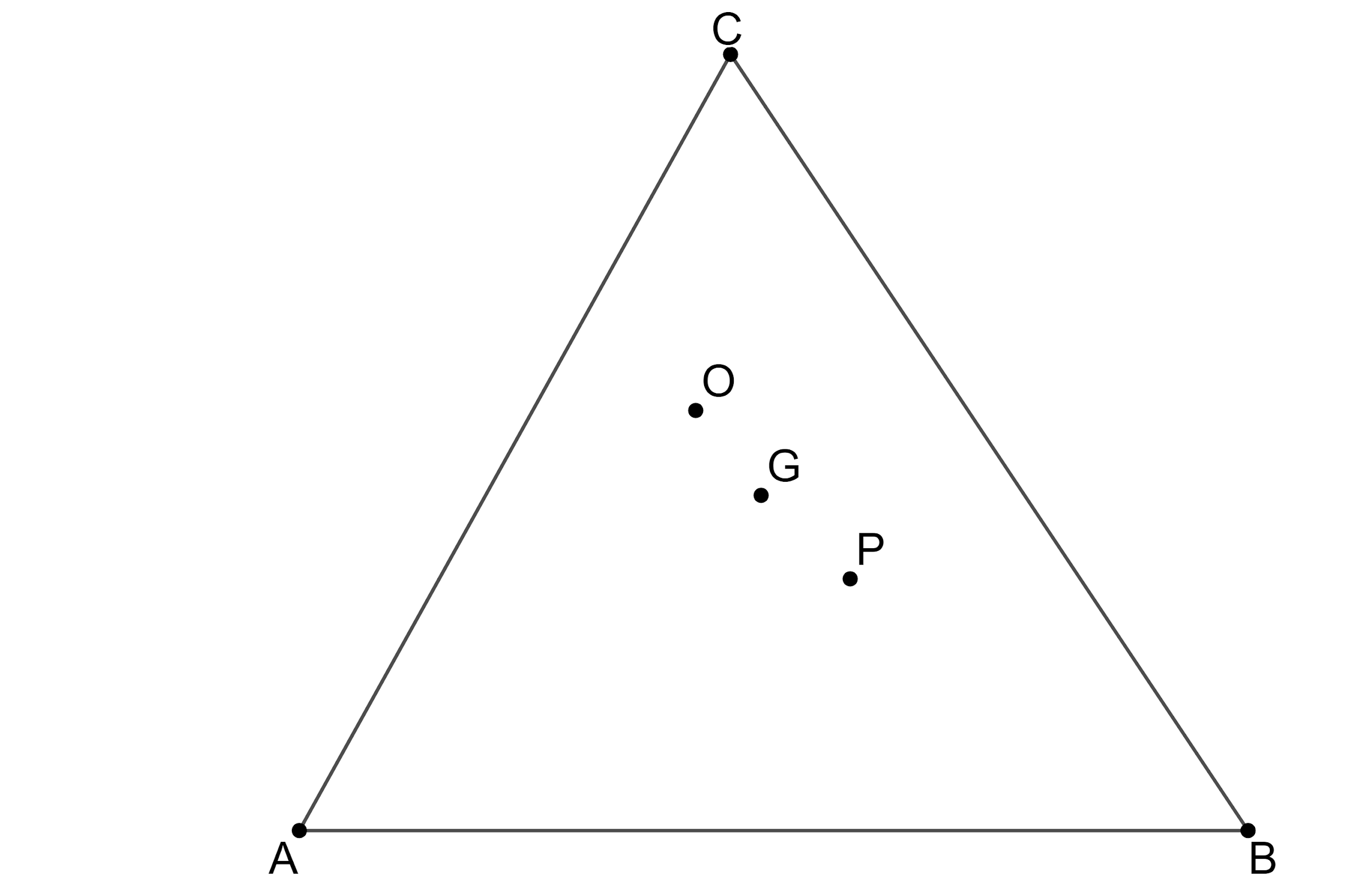
Let us assume ‘G’ be the centroid of the triangle ABC.
We know that the centroid of the triangle with position vectors of vertices
So, we get the position vector of ‘G’ as
We know that the centroid divided the line joining the orthocentre and circumcentre in a ratio of
Let us assume the orthocentre of the triangle is ‘O’.
We know that if a point S divides the points Q and R in the ratio of m:n internally, then
So, we get
Now, let us substitute
So, we have found the position of orthocentre of the triangle ABC as
Note:
We should confuse with the section formula as
Complete step by step answer:
According to the problem, we are given that ABC is a triangle whose circumcentre is at P and the position vectors of A, B, C and P are

Let us assume ‘G’ be the centroid of the triangle ABC.
We know that the centroid of the triangle with position vectors of vertices
So, we get the position vector of ‘G’ as
We know that the centroid divided the line joining the orthocentre and circumcentre in a ratio of
Let us assume the orthocentre of the triangle is ‘O’.
We know that if a point S divides the points Q and R in the ratio of m:n internally, then
So, we get
Now, let us substitute
So, we have found the position of orthocentre of the triangle ABC as
Note:
We should confuse with the section formula as
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What is the full form of POSCO class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

How do you prove that the diagonals of a rectangle class 10 maths CBSE




