
What is the limit as x approaches infinity of
Answer
449.7k+ views
Hint: Assume the required limit as L. Now, to find the value of
Complete step by step solution:
Here we have been asked to find the limit of the sine function as the domain value, i.e. x, tends to infinity.
Let us assume the limit value as L so mathematically we have,
As we know that infinity is not a real number so we cannot substitute
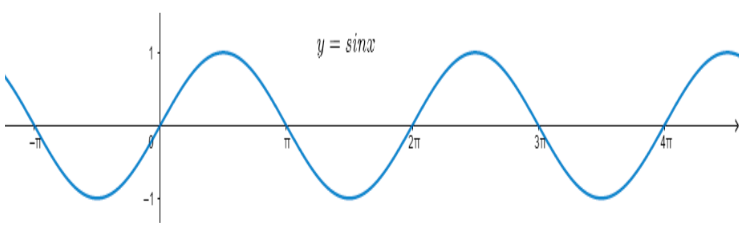
From the above graph we can clearly see that the value of sine function oscillates from -1 to +1. As we keep on increasing the value of x the value of
Hence, we can conclude that the limit does not exist.
Note: Here we do not have any other method to solve the question other than the graphical method because there is no any formula for the simplification of the above limit. You can also find the limits of other trigonometric functions using the similar approach if x is tending to infinity. Remember that the limit of the cosine and the tangent function is also non – existent at
Complete step by step solution:
Here we have been asked to find the limit of the sine function as the domain value, i.e. x, tends to infinity.
Let us assume the limit value as L so mathematically we have,
As we know that infinity is not a real number so we cannot substitute

From the above graph we can clearly see that the value of sine function oscillates from -1 to +1. As we keep on increasing the value of x the value of
Hence, we can conclude that the limit does not exist.
Note: Here we do not have any other method to solve the question other than the graphical method because there is no any formula for the simplification of the above limit. You can also find the limits of other trigonometric functions using the similar approach if x is tending to infinity. Remember that the limit of the cosine and the tangent function is also non – existent at
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




