Question

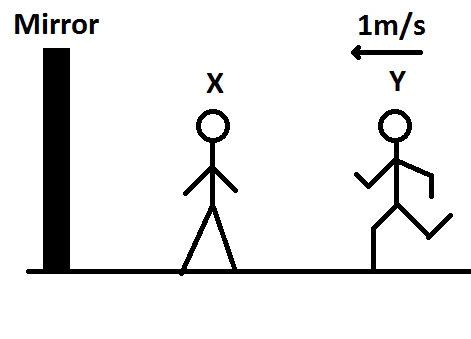
Man X is standing in front of the plane mirror while man Y is running towards him from behind. If the man Y is running at a speed of $ 1m\;{s^{ - 1}} $ , how many metres nearer does the manY seem to be away from man X after $ 5seconds $ ?

(A) $ 7m $
(B) $ 5m $
(C) $ 6m $
(D) $ 10m $

Answer 1

Hint :In this question, it is given that the man Y is running in front of the mirror. We know that in the case of the plane mirror, the object distance and the image distance will be the same. As the man X is stationary the velocity of the man Y with respect to him is the same as that with respect to the mirror.

Complete Step By Step Answer:
We know that for plane mirrors, the image distance is the same as the object distance but in the opposite direction.
Therefore, if we consider u as object distance and v as the image distance, we can say that
 $ \left| u \right| = \left| v \right| $
As per the definition of velocity, it is the distance covered during the unit time.
 $ \Rightarrow \left| {\dfrac{{du}}{{dt}}} \right| = \left| {\dfrac{{dv}}{{dt}}} \right| $
Thus, the speed of image is equal to the speed of man Y.
As the man X is stationary, Hence, the speed of man Y with respect to man X will be $ 1m\;{s^{ - 1}} $ .
Thus, we can say that the speed of image of man Y with respect to the man X is:
 $ 1 - \left( { - 1} \right) = 1 + 1 = 2m{s^{ - 1}} $
The distance covered by the image of man Y with respect to man X in $ 5seconds $ will be : $ 2 \times 5 = 10m $ .
Thus, the man Y seems to be $ 10m $ away from man X after $ 5seconds $ .
Hence, option D is the right answer.

Note :
Here, we have used the concept of the relative velocity. In the case of a plane mirror, we have seen that the velocities of the image and the object have the same magnitude. However the directions of these velocities are opposite.