
MI of a plane square lamina about an axis through its centre and parallel to one of its side is I. Then MI ‘I’ about an axis at an angle


Answer
494.1k+ views
Hint: To find the moment of inertia of any shape about an unknown axis, first we need to know the moment of inertia of the shape about an axis either perpendicular to it or parallel to it. If we know the moment of inertia of a shape about an axis passing through the centre of mass of the body, then we can find the moment of inertia about any axis parallel to it by the use of parallel axis theorem.
Complete answer:
Here, we are given the moment of inertia of the square about the axis ‘I’.
We can use this information to compute the moment of inertia about any axis which is similar to this one. Hence the moment of inertia about an axis in the plane of square and perpendicular to the given axis will also be ‘I’. Now, the moment of inertia of about an axis perpendicular to the plane is given by a perpendicular axis theorem.
Perpendicular axis theorem: Moment of inertia of a plane lamina about an axis perpendicular to the lamina is the sum of moment of inertia about two mutually perpendicular axis, lying in the lamina.
So,
But, the moment of inertia about an axis perpendicular to the plane will be the same for any two mutually perpendicular axes. Thus we have to choose one axis as given one and another perpendicular to it but in the plane, as shown.
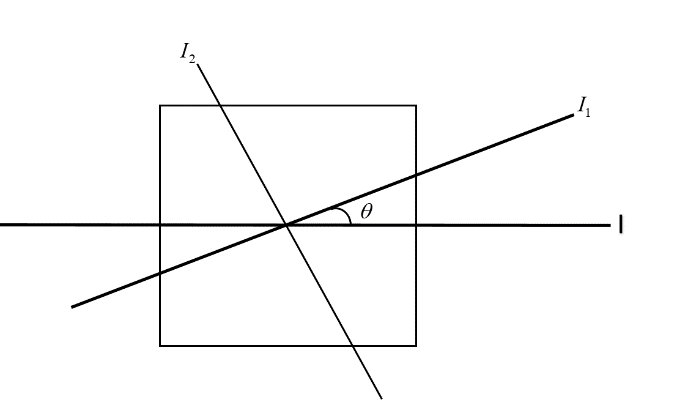
Hence
Now, as this case is of a square. Hence any axis passing through the centre and mutually perpendicular are alike.
Thus
Hence
Or
So, the correct answer is “Option A”.
Note:
One should note that we are liberal with the moment of inertias about different axes as the figure is symmetric. If the figure were not symmetrical (say rectangle), then we can’t go with this procedure and then the only tool that could be used is integration.
Complete answer:
Here, we are given the moment of inertia of the square about the axis ‘I’.
We can use this information to compute the moment of inertia about any axis which is similar to this one. Hence the moment of inertia about an axis in the plane of square and perpendicular to the given axis will also be ‘I’. Now, the moment of inertia of about an axis perpendicular to the plane is given by a perpendicular axis theorem.
Perpendicular axis theorem: Moment of inertia of a plane lamina about an axis perpendicular to the lamina is the sum of moment of inertia about two mutually perpendicular axis, lying in the lamina.
So,
But, the moment of inertia about an axis perpendicular to the plane will be the same for any two mutually perpendicular axes. Thus we have to choose one axis as given one and another perpendicular to it but in the plane, as shown.

Hence
Now, as this case is of a square. Hence any axis passing through the centre and mutually perpendicular are alike.
Thus
Hence
Or
So, the correct answer is “Option A”.
Note:
One should note that we are liberal with the moment of inertias about different axes as the figure is symmetric. If the figure were not symmetrical (say rectangle), then we can’t go with this procedure and then the only tool that could be used is integration.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




