
Moment of inertia of a thin rod of mass m and length l about at axis passing through a point
Answer
499.2k+ views
1 likes
Hint: Use the theorem of parallel axes. Substitute the values in the formula and get a moment of inertia of a thin rod of mass m and length l about at the axis passing through a point
Formula used:
Complete answer:
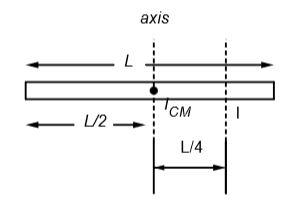
Given:
According to the theorem of parallel axes,
where, I: Moment of inertia of thin rod
But, we know
Therefore, substituting the values in the equation. (1) we get,
Therefore, Moment of inertia of a thin rod of mass m and length l about at axis passing through a point
So, the correct answer is “Option C”.
Note:
For a uniform rod with negligible thickness, the moment of inertia about its center of mass is
Formula used:
Complete answer:

Given:
According to the theorem of parallel axes,
where, I: Moment of inertia of thin rod
But, we know
Therefore, substituting the values in the equation. (1) we get,
Therefore, Moment of inertia of a thin rod of mass m and length l about at axis passing through a point
So, the correct answer is “Option C”.
Note:
For a uniform rod with negligible thickness, the moment of inertia about its center of mass is
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




