Question

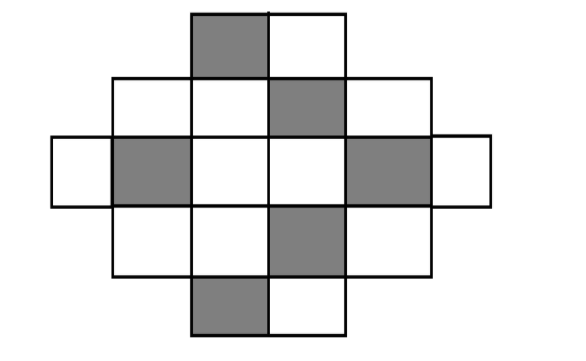
How many more squares in the figure must be shaded so that the fraction of the shaded squares is \[\dfrac{7}{9}\] ?

Answer 1

Hint: In order to determine the number of squares in the given figure, there are a total six shaded boxes. We can arrive at the solution by using the subtraction method i.e. subtract the six boxes from the required number of boxes to get the required fraction \[\dfrac{7}{9}\] .

Complete step-by-step answer:
A fraction is used to describe a component of something larger. It is a part of the whole representation in form of ratio. The numerator and denominator are the two elements of a fraction. The numerator is the number at the top, and the denominator is the number at the bottom.
We can solve the problem, the diagram given as follows:
In the given question, there are total \[18\] small squares. The number of shaded squares is \[6\] .
We require the shaded squares fraction to be \[\dfrac{7}{9}\] . Hence, we can find the total number of shades squares required as follows:
Let the number of shades squares be \[x\] .
 \[ \Rightarrow \dfrac{7}{9} = \dfrac{x}{{18}}\]
Cross-multiplying on the other side of equation, we get,
 \[ \Rightarrow x = \dfrac{7}{9} \times 18\]
Hence total number of shaded squares shall be:
 \[ \Rightarrow x = 14\]

We are already given that there are \[6\] shaded squares.
Hence remaining number of squares to be shaded are:
 \[ = 14 - 6\]
 \[ = 8\]
Hence \[8\] more squares need to be shaded so that the fraction of shaded squares is \[\dfrac{7}{9}\] .
So, the correct answer is “8”.

Note: The solution is based on the assumption that only the small squares are counted in the figure. The square formed by merging four boxes is not considered.
We can use the alternate method as follows:
There are \[6\] shaded squares out of \[18\] .
The current ratio of shaded square is \[\dfrac{6}{{18}} = \dfrac{1}{3}\] .
Let the number of squares to be shaded be \[x\] .
We require the ratio to be \[\dfrac{7}{9}\] so:
 \[\dfrac{1}{3} + \dfrac{x}{{18}} = \dfrac{7}{9}\]
Equalizing the denominators, we get,
 \[\dfrac{6}{{18}} + \dfrac{x}{{18}} = \dfrac{{14}}{{18}}\]
 \[\dfrac{{6 + x}}{{18}} = \dfrac{{14}}{{18}}\]
Dividing by \[18\] , we get,
 \[6 + x = 14\]
 \[x = 14 - 6 = 8\]
Hence, we require to shade \[8\] more squares.