
Number of lobes present in
(A) 10
(B) 5
(C) 4
(D) 2
Answer
495.3k+ views
Hint: To know the information about atomic orbital by referring to the quantum numbers are principal quantum number (n), angular quantum number (l), and magnetic quantum number (m). d- Orbital shape describes with the help of angular quantum number and magnetic quantum number which specifies the angular momentum of d-orbital.
Complete step by step solution:
The relation between the principal quantum number (n), angular quantum number (l), and magnetic quantum number (m) given as,
l= n-1, where n = 1, 2, 3 …
The values of m = -l….-3, -2, -1, 0, 1, 2, 3…+l
If n= 1, l=0, m= 0, the orbital represents s-orbital and only one sub orbital
If n = 2, l = 1, and m = -1, 0, +1, which represents p-orbital with three p- orbital are
For d- orbital, n= 3, l = 2 and m = +2, +1, 0, 1, 2, which represents five d-orbital
The shape of the
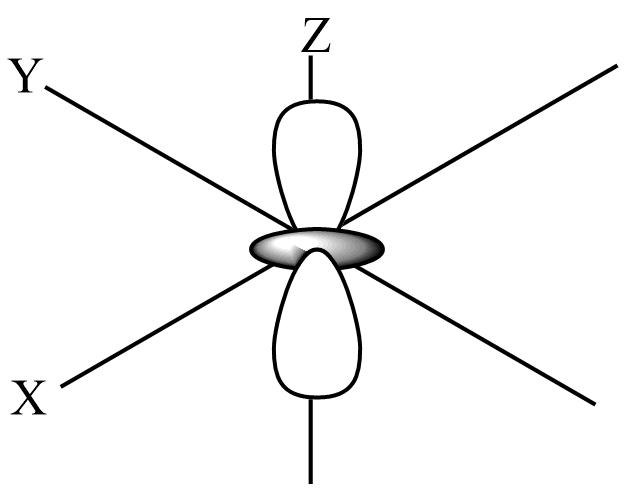
From the above shape of
Hence, the Number of lobes present in
The correct answer is option D.
Note: Generally, d-orbital has four lobes and 2 nodal planes. Except for
Complete step by step solution:
The relation between the principal quantum number (n), angular quantum number (l), and magnetic quantum number (m) given as,
l= n-1, where n = 1, 2, 3 …
The values of m = -l….-3, -2, -1, 0, 1, 2, 3…+l
If n= 1, l=0, m= 0, the orbital represents s-orbital and only one sub orbital
If n = 2, l = 1, and m = -1, 0, +1, which represents p-orbital with three p- orbital are
For d- orbital, n= 3, l = 2 and m = +2, +1, 0, 1, 2, which represents five d-orbital
The shape of the

From the above shape of
Hence, the Number of lobes present in
The correct answer is option D.
Note: Generally, d-orbital has four lobes and 2 nodal planes. Except for
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




