Question

Number of rectangles in figure shown which are not squares are is:

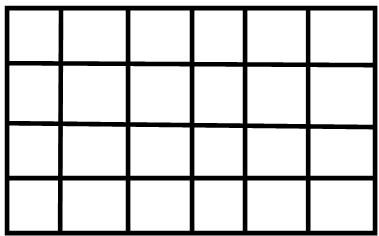
A) 159
B) 160
C) 161
D) None of these

Answer 1

Hint:
Here we need to find the number of rectangles in a given grid. Here we will first count the number of rows and columns in the grid. We will use the formula to find the number of rectangles in a grid. Then we will use the formula to find the number of squares in a grid. We need to find the number of rectangles which are not squares, for that we will subtract the number of squares from the number of rectangles to get the required number of rectangles.

Formula Used: We will use the following formulas:
Number of rectangles in a grid \[ = \left( {\dfrac{{m + 1}}{2}} \right)m \times \left( {\dfrac{{n + 1}}{2}} \right)n\] , where \[m\] is the number of rows and \[n\] is the number of columns.
Number of squares in a grid \[ = m \times n + \left( {m - 1} \right)\left( {n - 1} \right) + \left( {m - 2} \right)\left( {n - 2} \right) + \left( {m - 3} \right)\left( {n - 3} \right) + ....\], where \[m\] is the number of rows and \[n\] is the number of columns.

Complete step by step solution:
The number of rows in a grid is 4 and number of columns in a grid is 6. i.e \[m = 4\] and \[n = 6\].
Putting \[m = 4\] and \[n = 6\] in the formula \[\left( {\dfrac{{m + 1}}{2}} \right)m \times \left( {\dfrac{{n + 1}}{2}} \right)n\], we get
 Number of rectangles in a grid \[ = \left( {\dfrac{{4 + 1}}{2}} \right)4 \times \left( {\dfrac{{6 + 1}}{2}} \right)6\]
Simplifying the terms further, we get
Number of rectangles in a grid \[ = \dfrac{5}{2} \times 4 \times \dfrac{7}{2} \times 6\]
After multiplying the terms, we get
Number of rectangles in a grid \[ = 210\]
Now, we will find the number of squares in a grid.
Putting \[m = 4\] and \[n = 6\] in the formula \[m \times n + \left( {m - 1} \right)\left( {n - 1} \right) + \left( {m - 2} \right)\left( {n - 2} \right) + \left( {m - 3} \right)\left( {n - 3} \right) + ....\], we get
Number of squares in a grid \[ = 4 \times 6 + \left( {4 - 1} \right)\left( {6 - 1} \right) + \left( {4 - 2} \right)\left( {6 - 2} \right) + \left( {4 - 3} \right)\left( {6 - 3} \right)\]
Simplifying the terms further, we get
Number of squares in a grid \[ = 4 \times 6 + 3 \times 5 + 2 \times 4 + 1 \times 3\]
After multiplying the then adding the terms, we get
Number of squares in a grid \[ = 50\]
But we have to find the number of rectangles which are not squares.
Therefore, the required number of rectangles which are not squares is equal to the difference between the number of rectangles and number of squares.

Required number of rectangles \[ = 210 - 50 = 160\].

Note:
Here we have calculated the number of rectangles in the grid. A grid is defined as a network which is formed by the intersection of parallel lines, the lines may be real and imaginary and due to the intersection of parallel lines, rectangles and squares are formed.