
Obtain the relation between the magnitude of linear acceleration and angular acceleration in circular motion.
Answer
487.2k+ views
Hint: As a first step, you could find the relation between the linear and angular velocity. Then you could recall the definitions of linear acceleration and angular acceleration. Inter-relating the above relation you will get the required relation between linear and angular accelerations. Also, the remember radius remains constant in circular motion.
Formula used:
Linear velocity,
Linear acceleration,
Angular acceleration,
Complete step by step solution:
In the question, we are asked to obtain the relation between the magnitudes of linear acceleration and that of angular acceleration in the case of circular motion.
For a uniform circular motion we know that the motion is in a circle with constant speed and hence constant angular velocity.
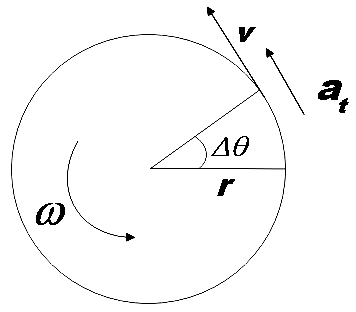
Angular velocity
We also have the relation between angular velocity
Where r is the radius of curvature of the circle in which the motion takes place.
Angular acceleration
The unit of angular acceleration is
Now let us discuss the relation of linear and angular acceleration.
The linear acceleration is found to be tangential to the circle at a particular point for circular motion and hence is called tangential acceleration
As the linear acceleration is directly proportional to time rate of velocity,
From (2),
But the radius remains constant in circular motion.
From (3),
Therefore, we find that linear acceleration is directly proportional to angular acceleration.
Note: From the final expression we see that, the higher the magnitude of angular acceleration is, the higher the magnitude of linear acceleration will be. Let us consider the real life example of a car. Greater angular acceleration of the car’s wheels implies greater acceleration of the car. Also, for a particular value of angular acceleration, the smaller the wheels of the car is, the smaller will be its linear acceleration.
Formula used:
Linear velocity,
Linear acceleration,
Angular acceleration,
Complete step by step solution:
In the question, we are asked to obtain the relation between the magnitudes of linear acceleration and that of angular acceleration in the case of circular motion.
For a uniform circular motion we know that the motion is in a circle with constant speed and hence constant angular velocity.

Angular velocity
We also have the relation between angular velocity
Where r is the radius of curvature of the circle in which the motion takes place.
Angular acceleration
The unit of angular acceleration is
Now let us discuss the relation of linear and angular acceleration.
The linear acceleration is found to be tangential to the circle at a particular point for circular motion and hence is called tangential acceleration
As the linear acceleration is directly proportional to time rate of velocity,
From (2),
But the radius remains constant in circular motion.
From (3),
Therefore, we find that linear acceleration is directly proportional to angular acceleration.
Note: From the final expression we see that, the higher the magnitude of angular acceleration is, the higher the magnitude of linear acceleration will be. Let us consider the real life example of a car. Greater angular acceleration of the car’s wheels implies greater acceleration of the car. Also, for a particular value of angular acceleration, the smaller the wheels of the car is, the smaller will be its linear acceleration.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




