
On an open ground a motorist follows a track that turns to his left by an angle of
Answer
481.5k+ views
1 likes
Hint: Displacement as defined as the difference between the initial and the final position between the two points and distance is defined as the amount of length covered by the person in reach of a particular place. Here, imagine a motorcyclist taking
Complete step by step solution:
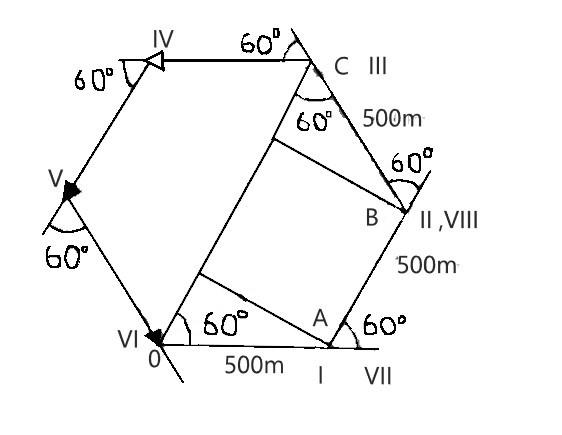
Step 1:
See the above diagram, in this diagram the magnitude of length at the 3rd turn:
Put the value of the length in the above relation
Dot the necessary calculation:
The above value is displacement of the motorcyclist at the 3rd turn for the displacement:
Now, the ratio between the displacement and distance is:
Step 2:
At 6th turn: The initial position is same as the final position so the displacement and distance will be:
Now, the ratio between them is:
Step 3:
At the 8th turn the displacement and the distance would be:
Multiply and divide by “2” on the LHS:
Do the necessary calculation:
The ratio between the two would be:
Final Answer: The displacement at the third, sixth and eight turn of the motorcyclist is 1000m,0m and
Note: Here, the question is not that more complex than it appears to be. We need to resolve the angle and add them together to get to the asked turn. Distance is a vector quantity so we need to resolve the angle just add the distances together.
Complete step by step solution:

Step 1:
See the above diagram, in this diagram the magnitude of length at the 3rd turn:
Put the value of the length in the above relation
Dot the necessary calculation:
The above value is displacement of the motorcyclist at the 3rd turn for the displacement:
Now, the ratio between the displacement and distance is:
Step 2:
At 6th turn: The initial position is same as the final position so the displacement and distance will be:
Now, the ratio between them is:
Step 3:
At the 8th turn the displacement and the distance would be:
Multiply and divide by “2” on the LHS:
Do the necessary calculation:
The ratio between the two would be:
Final Answer: The displacement at the third, sixth and eight turn of the motorcyclist is 1000m,0m and
Note: Here, the question is not that more complex than it appears to be. We need to resolve the angle and add them together to get to the asked turn. Distance is a vector quantity so we need to resolve the angle just add the distances together.
Watch videos on
On an open ground a motorist follows a track that turns to his left by an angle of

Motion in a Plane class 11 Physics - NCERT EXERCISE 3.10 | Physics NCERT | Gaurav Tiwari
Subscribe
 Share
Share likes
7.3K Views
1 year ago
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
EMI starts from ₹3,487.34 per month
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE



 Watch Video
Watch Video
