
One mole of an ideal gas at pressure
A.
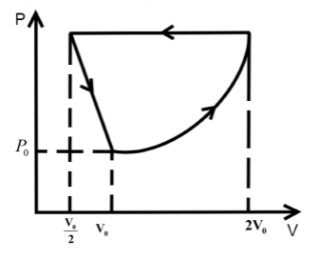
B.
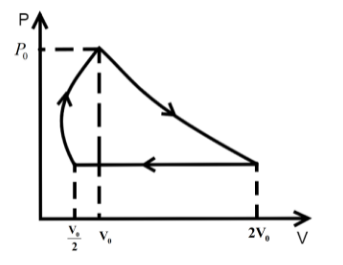
C.
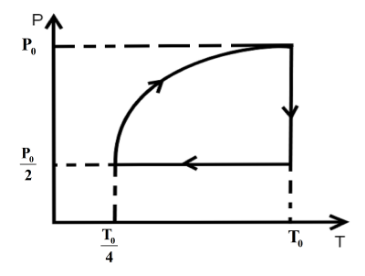
D.





Answer
428.7k+ views
Hint:In an isothermal process the temperature of the reaction is kept constant and the P-T or V-T curve is straight parallel to the T axis. For an isobaric process the pressure of the reaction is kept constant and the P-V or P-T curve is straight parallel to the P axis.
Formula used:
Boyle’s law for ideal gas is given by,
where
Charles’s law for ideal gas is given by,
where,
Complete step by step answer:
We know that in an isothermal process the temperature of the reaction is kept constant while other parameters of the equation of state vary .So, for an isothermal process the P-T or V-T curve is straight parallel to the T axis. Here, it is expanded to keep the temperature constant. So, from Boyle’s law we can get the final pressure as,
In the second step the pressure is kept constant and we know for an isobaric process the pressure of the reaction is kept constant while other parameters of the equation of state vary. So, the P-V or P-T curve is straight parallel to the P axis. So, from Charles law we can get final temperature in the process as,
In the third step the
So, the state parameter of the gas changes as,
So, the P-T curve can be drawn as,
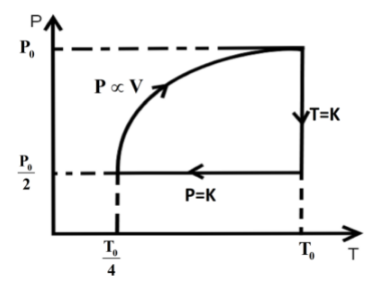
Hence, option C is the correct answer.
Note:To draw the curve, always deduce the state parameters
Formula used:
Boyle’s law for ideal gas is given by,
where
Charles’s law for ideal gas is given by,
where,
Complete step by step answer:
We know that in an isothermal process the temperature of the reaction is kept constant while other parameters of the equation of state vary .So, for an isothermal process the P-T or V-T curve is straight parallel to the T axis. Here, it is expanded to keep the temperature constant. So, from Boyle’s law we can get the final pressure as,
In the second step the pressure is kept constant and we know for an isobaric process the pressure of the reaction is kept constant while other parameters of the equation of state vary. So, the P-V or P-T curve is straight parallel to the P axis. So, from Charles law we can get final temperature in the process as,
In the third step the
So, the state parameter of the gas changes as,
So, the P-T curve can be drawn as,

Hence, option C is the correct answer.
Note:To draw the curve, always deduce the state parameters
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Why is steel more elastic than rubber class 11 physics CBSE

Explain why a There is no atmosphere on the moon b class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE




