
Ordinate of all points on X-axis is
Answer
474.9k+ views
Hint: The given question is about to write the ordinates of all the points on
Complete step-by-step answer:
The question is to write the ordinates of all the points on
Now In the given question, We have to write the ordinates of all the points on
Let us clear it by making
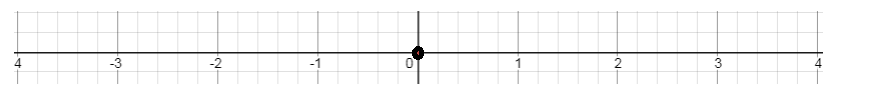
Since on
Hence There are zero ordinates of all the points on
Note: There are zero ordinates of all the points on
Complete step-by-step answer:
The question is to write the ordinates of all the points on
Now In the given question, We have to write the ordinates of all the points on
Let us clear it by making

Since on
Hence There are zero ordinates of all the points on
Note: There are zero ordinates of all the points on
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

The southernmost point of the Indian mainland is known class 7 social studies CBSE

Convert 200 Million dollars in rupees class 7 maths CBSE

How many crores make 10 million class 7 maths CBSE

Write a pair of integers whose sum gives i Zero ii class 7 maths CBSE

List of coprime numbers from 1 to 100 class 7 maths CBSE





