
P \[\left( -1,4 \right)\], Q \[\left( 11,-8 \right)\] divides AB harmonically in the ratio \[3:2\] then A,B are?
Answer
436.5k+ views
Hint:Using the section formula method we first multiply the \[x\] coordinates with the ratio \[3:2\] and then do the same for \[y\] coordinates as well.
For \[x\] coordinates and coordinates of A and B as \[\left( a,b \right)\] and \[\left( x,y \right)\] with ratio
\[\left( m:n \right)\]:
\[\dfrac{mx+na}{m+n}\]
For \[y\] coordinates and coordinates of A and B as \[\left( a,b \right)\] and \[\left( x,y \right)\] with ratio\[\left( m:n \right)\]:
\[\dfrac{my+nb}{m+n}\]
The previous formula was applied for P coordinate and now we will do the same for Q coordinates as well. The ratio of \[3:2\] will change to \[-3:2\] as Q is a harmonic conjugate of P.
Complete step by step solution:
Now as given in the question, we first form a coordinate diagram where P is the midpoint with A, B as extreme and the distance of AP is 3 and the distance of PB is 2.
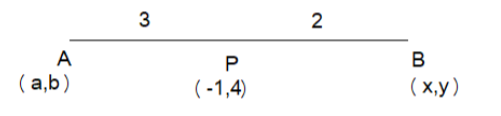
After this let us form an equation with the help of A's coordinate and B's coordinate with midpoint as
\[\left( -1,4 \right)\].
The equation for the \[a\] or \[x\] coordinate is given as:
\[\Rightarrow \dfrac{3\times x+2\times a}{3+2}=-1\]
\[\Rightarrow 3x+2a=-5\] …(1)
The equation for the \[b\] or \[y\] coordinate is given as:
\[\Rightarrow \dfrac{3\times y+2\times b}{3+2}=4\]
\[\Rightarrow 3y+2b=20\] …(2)
We now find equation for \[x\] and \[y\] with midpoint being \[\left( 11,-8 \right)\] and the ratio of distance from AQ to QB as \[\left( -3:2 \right)\] as Q is harmonic conjugate of P.
The equation for the \[a\] or \[x\] coordinate is given as:
\[\Rightarrow \dfrac{-3\times x+2\times a}{-3+2}=11\]
\[\Rightarrow -3x+2a=-11\] …(3)
The equation for the \[b\] or \[y\] coordinate is given as:
\[\Rightarrow \dfrac{-3\times y+2\times b}{-3+2}=8\]
\[\Rightarrow -3y+2b=8\] …(4)
Now we equate the Equation 1, 2, 3 and 4; So as to find the value of a, b. First we find for the value of a by equating equation 1,3.
\[\begin{align}
& \text{ }3x+2a=-5 \\
& -3x+2a=-11 \\
& \text{ +}4a=-16 \\
\end{align}\]
\[\Rightarrow a=-4\]
We get the value of \[a\] as \[-4\] and to find the value of \[x\] we place the value of \[a\] in \[3x+2a= -5\].
\[\Rightarrow 3x+2\times -4=-5\]
\[\Rightarrow 3x=8-5\]
\[\Rightarrow x=1\]
Then we find for the value of b by Equating equation 2,4.
\[\begin{align}
& \text{ }3y+2b=20 \\
& -3y+2b=8 \\
& \text{ +}4b=28 \\
\end{align}\]
\[\Rightarrow b=7\]
We get the value of \[b\] as \[7\] and to find the value of \[x\] we place the value of \[b\] in \[-3y+2b=8\].
\[\Rightarrow -3y+2\times 7=8\]
\[\Rightarrow -3y=-14+8\]
\[\Rightarrow y=2\]
Therefore, the value of a, b or A, B is given as \[\left( -4:7 \right)\]
Note: The term harmonic conjugate means that if the line is divided let say in ratio of \[\text{AC:BC = AD:BD}\] we can say that C and D are cutting the line AB harmonically and that AB and CD are harmonic conjugates.

For \[x\] coordinates and coordinates of A and B as \[\left( a,b \right)\] and \[\left( x,y \right)\] with ratio
\[\left( m:n \right)\]:
\[\dfrac{mx+na}{m+n}\]
For \[y\] coordinates and coordinates of A and B as \[\left( a,b \right)\] and \[\left( x,y \right)\] with ratio\[\left( m:n \right)\]:
\[\dfrac{my+nb}{m+n}\]
The previous formula was applied for P coordinate and now we will do the same for Q coordinates as well. The ratio of \[3:2\] will change to \[-3:2\] as Q is a harmonic conjugate of P.
Complete step by step solution:
Now as given in the question, we first form a coordinate diagram where P is the midpoint with A, B as extreme and the distance of AP is 3 and the distance of PB is 2.
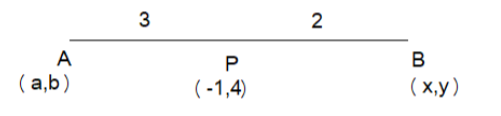
After this let us form an equation with the help of A's coordinate and B's coordinate with midpoint as
\[\left( -1,4 \right)\].
The equation for the \[a\] or \[x\] coordinate is given as:
\[\Rightarrow \dfrac{3\times x+2\times a}{3+2}=-1\]
\[\Rightarrow 3x+2a=-5\] …(1)
The equation for the \[b\] or \[y\] coordinate is given as:
\[\Rightarrow \dfrac{3\times y+2\times b}{3+2}=4\]
\[\Rightarrow 3y+2b=20\] …(2)
We now find equation for \[x\] and \[y\] with midpoint being \[\left( 11,-8 \right)\] and the ratio of distance from AQ to QB as \[\left( -3:2 \right)\] as Q is harmonic conjugate of P.
The equation for the \[a\] or \[x\] coordinate is given as:
\[\Rightarrow \dfrac{-3\times x+2\times a}{-3+2}=11\]
\[\Rightarrow -3x+2a=-11\] …(3)
The equation for the \[b\] or \[y\] coordinate is given as:
\[\Rightarrow \dfrac{-3\times y+2\times b}{-3+2}=8\]
\[\Rightarrow -3y+2b=8\] …(4)
Now we equate the Equation 1, 2, 3 and 4; So as to find the value of a, b. First we find for the value of a by equating equation 1,3.
\[\begin{align}
& \text{ }3x+2a=-5 \\
& -3x+2a=-11 \\
& \text{ +}4a=-16 \\
\end{align}\]
\[\Rightarrow a=-4\]
We get the value of \[a\] as \[-4\] and to find the value of \[x\] we place the value of \[a\] in \[3x+2a= -5\].
\[\Rightarrow 3x+2\times -4=-5\]
\[\Rightarrow 3x=8-5\]
\[\Rightarrow x=1\]
Then we find for the value of b by Equating equation 2,4.
\[\begin{align}
& \text{ }3y+2b=20 \\
& -3y+2b=8 \\
& \text{ +}4b=28 \\
\end{align}\]
\[\Rightarrow b=7\]
We get the value of \[b\] as \[7\] and to find the value of \[x\] we place the value of \[b\] in \[-3y+2b=8\].
\[\Rightarrow -3y+2\times 7=8\]
\[\Rightarrow -3y=-14+8\]
\[\Rightarrow y=2\]
Therefore, the value of a, b or A, B is given as \[\left( -4:7 \right)\]
Note: The term harmonic conjugate means that if the line is divided let say in ratio of \[\text{AC:BC = AD:BD}\] we can say that C and D are cutting the line AB harmonically and that AB and CD are harmonic conjugates.

Recently Updated Pages
Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
10 examples of friction in our daily life

What problem did Carter face when he reached the mummy class 11 english CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

State and prove Bernoullis theorem class 11 physics CBSE

The sequence of spore production in Puccinia wheat class 11 biology CBSE




