
What is the parameter of a parallelogram with sides
A)
B)
C)
D)
Answer
495.9k+ views
Hint: Parallelogram is a type of quadrilateral that has equal and parallel opposite sides. So, to solve this problem we will first draw a figure where length of parallelogram will be equals to
Complete step-by-step answer:
We have been given a parallelogram with sides
So, let us draw a figure of a parallelogram using above details, i.e., length of parallelogram will be equals to
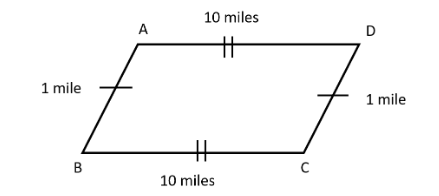
Since, we know that parallelogram has equal and parallel opposite sides, so here side AB is parallel to side CD and side AD is parallel to side BC. Also,
Now, we know that, parameter of parallelogram
On putting the values in the above formula, we get
So, the parameter of parallelogram is
Thus, option (B)
So, the correct answer is “Option B”.
Note: Let us know some facts or important properties about parallelograms. So, opposite sides of parallelograms are congruent. And opposite angles are also congruent. The consecutive angles of parallelogram are supplementary, i.e., equals to
Complete step-by-step answer:
We have been given a parallelogram with sides
So, let us draw a figure of a parallelogram using above details, i.e., length of parallelogram will be equals to

Since, we know that parallelogram has equal and parallel opposite sides, so here side AB is parallel to side CD and side AD is parallel to side BC. Also,
Now, we know that, parameter of parallelogram
On putting the values in the above formula, we get
So, the parameter of parallelogram is
Thus, option (B)
So, the correct answer is “Option B”.
Note: Let us know some facts or important properties about parallelograms. So, opposite sides of parallelograms are congruent. And opposite angles are also congruent. The consecutive angles of parallelogram are supplementary, i.e., equals to
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Questions & Answers - Ask your doubts

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Science: Engaging Questions & Answers for Success

Trending doubts
List some examples of Rabi and Kharif crops class 8 biology CBSE

What is the feminine gender of a stag class 8 english CBSE

Write five sentences about Earth class 8 biology CBSE

How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE





