Question

How perimeter of segment of a circle is \[\dfrac{{\pi r\theta }}{{{{180}^ \circ }}} + 2r\sin \left( {\dfrac{\theta }{2}} \right)\]. Give derivation.

Answer 1

Hint: We will first of all create a circle of radius r and a sector. Then join the end points of the sector by a line to form a chord and thus a segment. Now, to find the perimeter of the segment you just need to add the length of the chord and the arc length.

Complete step-by-step answer:
Let us first draw a circle with radius r and a sector with angle \[\angle AOB = \theta \] and then by joining the end points A and B of the sector, we will get a chord AB as given below:

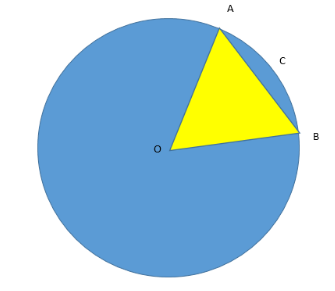
Here, O is the center, AB is the chord and C is a point on the arc ACB.
Now, without loss of generality, let us say we have the segment ACB to find the perimeter of.
To find its perimeter, we definitely need to add the length of the arc and the length of the chord as well.
We know that the length of an arc with angle \[\theta \] is given by $\dfrac{\theta }{{{{360}^ \circ }}} \times 2\pi r = \dfrac{{\theta \pi r}}{{{{180}^ \circ }}}$……….(1)
Now, let us try to find the length of the chord AB. Draw a perpendicular from point O to AB.

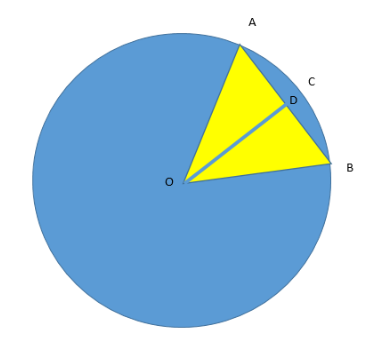
Since, we know that we have a theorem: Perpendicular from center to chord bisects the chord.
Hence, AD = DB. ………………(2)
Now, consider $\vartriangle AOD$ and $\vartriangle BOD$:
AD = DB (Using 2)
OD = OD (Common)
OA = OB (Radii of same circle)
Hence, $\vartriangle AOD \cong \vartriangle BOD$ (By SSS property rule)
[SSS Rule: If three sides of one triangle are equal to three sides of another triangle, the triangles are congruent]
Hence, $\angle AOD = \angle BOD$ (By CPCT)
[CPCT stands for Corresponding parts of congruent triangles. CPCT theorem states that if two or more triangles which are congruent to each other are taken then the corresponding angles and the sides of the triangles are also congruent to each other]
Hence, if \[\angle AOB = \theta \], then $\angle AOD = \angle BOD = \dfrac{\theta }{2}$.
Now, in $\vartriangle AOD$, we have $\sin \left( {\dfrac{\theta }{2}} \right) = \dfrac{{AD}}{{OA}}$
On cross multiplying and simplifying and putting in OA = r, we get:-
$ \Rightarrow AD = r\sin \left( {\dfrac{\theta }{2}} \right)$
Now, using (2), we get: $AD = DB = r\sin \left( {\dfrac{\theta }{2}} \right)$
Adding both, we get:-
$ \Rightarrow AD + DB = 2r\sin \left( {\dfrac{\theta }{2}} \right)$
We can rewrite it as:-
$ \Rightarrow AB = 2r\sin \left( {\dfrac{\theta }{2}} \right)$ ……………(3)
Adding both (1) and (3) to get the perimeter:-
Perimeter of segment ADBC = $\dfrac{{\theta \pi r}}{{{{180}^ \circ }}} + 2r\sin \left( {\dfrac{\theta }{2}} \right)$.
Hence, proved.

Note: The students must note that the arc length formula, we just wrote can be visualized as well instead of learning only. Since, everyone knows that the circumference of a circle is given by $2\pi r$ .
We also know that the angle in a circle is ${360^ \circ }$.
It means for ${360^ \circ }$, the boundary is $2\pi r$.
So, for ${1^ \circ }$, the boundary is given by $\dfrac{{2\pi r}}{{{{360}^ \circ }}}$.
Hence, for $\theta $, the boundary will be $\dfrac{{2\pi r}}{{{{360}^ \circ }}} \times \theta = \dfrac{{\pi r\theta }}{{{{180}^ \circ }}}$.