Answer
396k+ views
Hint: First of all find the length of the side CD. To find the length of the side CE consider ABCE as a rectangle and use the fact that the opposite sides of a rectangle are equal and use the relations CE = AB and BC = AE. To find the length of the side DE consider AD as hypotenuse (H), DE as the base (B) and AE as the perpendicular (P) of the right angle triangle AED. Use the Pythagoras theorem given as ${{H}^{2}}={{P}^{2}}+{{B}^{2}}$ to find the value of DE. Add CE and DE to find the length of CD and finally take the sum of the length of all the sides of the trapezium ABCD to get the answer.
Complete step by step solution:
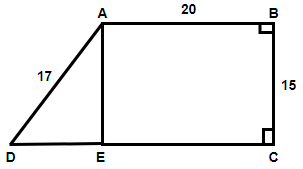
Here we have been provided with a trapezium with the following measurements shown below. We are asked to calculate the perimeter of the trapezium. First we need to find the length of the fourth side that is CD.
Now, clearly we can consider ABCE as a rectangle and we know that the opposite sides of a rectangle are equal so we have the following relations: -
$\Rightarrow $ AE = BC = 15 ……. (1)
$\Rightarrow $ AB = CE = 20 ……. (2)
Now, in the right angle triangle AED we have AD as hypotenuse (H), DE as the base (B) and AE as the perpendicular (P). Therefore, using the Pythagoras theorem given as ${{H}^{2}}={{P}^{2}}+{{B}^{2}}$ we get,
$\Rightarrow {{\left( AD \right)}^{2}}={{\left( AE \right)}^{2}}+{{\left( DE \right)}^{2}}$
Substituting the values AD = 17 and AE = 15 {relation (1)} we get,
$\begin{align}
& \Rightarrow {{\left( 17 \right)}^{2}}={{\left( 15 \right)}^{2}}+{{\left( DE \right)}^{2}} \\
& \Rightarrow {{\left( DE \right)}^{2}}=289-225 \\
& \Rightarrow {{\left( DE \right)}^{2}}=64 \\
\end{align}$
Taking square root both the sides we get,
$\Rightarrow $ DE = 8
Therefore the total length of the side CD is sum of the length of the sides CE and DE, so we get,
$\Rightarrow $ CD = CE + DE
Substituting the obtained value of DE and using relation (2) for the value of CE we get,
$\Rightarrow $ CD = 20 + 8
$\Rightarrow $ CD = 28
Now, the perimeter of the trapezium ABCD is the sum of the length of all the sides of the trapezium, so we have,
$\Rightarrow $ Perimeter = AB + BC + CD + DA
$\Rightarrow $ Perimeter = 20 + 15 + 28 + 17
$\therefore $ Perimeter = 80
So, the correct answer is “Option d”.
Note: You must remember the properties of certain special quadrilaterals like parallelogram, square, rectangle, rhombus etc. as any of their properties may be used in the questions where we need to find the area or the perimeter of a figure. Also remember the formula for the area of a trapezium given as $\dfrac{1}{2}\times $ sum of parallel sides $\times $ perpendicular distance between the parallel sides.
Complete step by step solution:
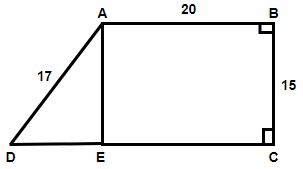
Here we have been provided with a trapezium with the following measurements shown below. We are asked to calculate the perimeter of the trapezium. First we need to find the length of the fourth side that is CD.

Now, clearly we can consider ABCE as a rectangle and we know that the opposite sides of a rectangle are equal so we have the following relations: -
$\Rightarrow $ AE = BC = 15 ……. (1)
$\Rightarrow $ AB = CE = 20 ……. (2)
Now, in the right angle triangle AED we have AD as hypotenuse (H), DE as the base (B) and AE as the perpendicular (P). Therefore, using the Pythagoras theorem given as ${{H}^{2}}={{P}^{2}}+{{B}^{2}}$ we get,
$\Rightarrow {{\left( AD \right)}^{2}}={{\left( AE \right)}^{2}}+{{\left( DE \right)}^{2}}$
Substituting the values AD = 17 and AE = 15 {relation (1)} we get,
$\begin{align}
& \Rightarrow {{\left( 17 \right)}^{2}}={{\left( 15 \right)}^{2}}+{{\left( DE \right)}^{2}} \\
& \Rightarrow {{\left( DE \right)}^{2}}=289-225 \\
& \Rightarrow {{\left( DE \right)}^{2}}=64 \\
\end{align}$
Taking square root both the sides we get,
$\Rightarrow $ DE = 8
Therefore the total length of the side CD is sum of the length of the sides CE and DE, so we get,
$\Rightarrow $ CD = CE + DE
Substituting the obtained value of DE and using relation (2) for the value of CE we get,
$\Rightarrow $ CD = 20 + 8
$\Rightarrow $ CD = 28
Now, the perimeter of the trapezium ABCD is the sum of the length of all the sides of the trapezium, so we have,
$\Rightarrow $ Perimeter = AB + BC + CD + DA
$\Rightarrow $ Perimeter = 20 + 15 + 28 + 17
$\therefore $ Perimeter = 80
So, the correct answer is “Option d”.
Note: You must remember the properties of certain special quadrilaterals like parallelogram, square, rectangle, rhombus etc. as any of their properties may be used in the questions where we need to find the area or the perimeter of a figure. Also remember the formula for the area of a trapezium given as $\dfrac{1}{2}\times $ sum of parallel sides $\times $ perpendicular distance between the parallel sides.
Recently Updated Pages
Fill in the blanks with suitable prepositions Break class 10 english CBSE

Fill in the blanks with suitable articles Tribune is class 10 english CBSE

Rearrange the following words and phrases to form a class 10 english CBSE

Select the opposite of the given word Permit aGive class 10 english CBSE

Fill in the blank with the most appropriate option class 10 english CBSE

Some places have oneline notices Which option is a class 10 english CBSE

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

How do you graph the function fx 4x class 9 maths CBSE

Which are the Top 10 Largest Countries of the World?

What is the definite integral of zero a constant b class 12 maths CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Define the term system surroundings open system closed class 11 chemistry CBSE

Full Form of IASDMIPSIFSIRSPOLICE class 7 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE