
Prove by the vector method ,the law of sine in trigonometry:

Answer
504.3k+ views
Hint: For solving this question we will assume that
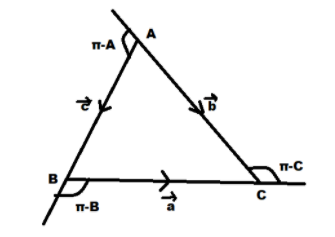
For a triangle ABC ,
Then just solve the question by using the cross product/ vector product of vectors method to get the desired answer.
The magnitude of cross product of two vectors is equal to the product of magnitude of both the vectors and the sine of angle between them.
Where
Complete step-by-step answer:
Let us assume
Now as we know that for a triangle
Since we know that:
Hence putting the value we get:-
Hence putting the respective values we get:-
Using cross product of vectors method, multiply by
Now as we know that:
Applying this formula we get:-
We know that the cross product of same vectors is zero
Therefore,
Putting this value we get:-
Now as we know that the magnitude of cross product of two vectors is equal to the product of magnitude of both the vectors and the sine of angle between them.
Where
Hence applying this formula for both LHS and RHS we get:-
Since it is given that the angle between vectors a and b is
Therefore substituting the values in above formula we get:-
Now since we know that
Hence we get:-
Similarly, we can prove that
Hence from equations 1 and 2 we get:-
Hence proved
Note: In this type of question students should first analyse the diagram instead of solving the equations. All the vectors should be taken according to their directions.
Also while calculating the magnitude of the vector product, students should take the correct angle between the two vectors.
For a triangle ABC ,
Then just solve the question by using the cross product/ vector product of vectors method to get the desired answer.
The magnitude of cross product of two vectors is equal to the product of magnitude of both the vectors and the sine of angle between them.
Where
Complete step-by-step answer:
Let us assume
Now as we know that for a triangle
Since we know that:
Hence putting the value we get:-
Hence putting the respective values we get:-
Using cross product of vectors method, multiply by
Now as we know that:
Applying this formula we get:-
We know that the cross product of same vectors is zero
Therefore,
Putting this value we get:-
Now as we know that the magnitude of cross product of two vectors is equal to the product of magnitude of both the vectors and the sine of angle between them.
Where
Hence applying this formula for both LHS and RHS we get:-
Since it is given that the angle between vectors a and b is
Therefore substituting the values in above formula we get:-
Now since we know that
Hence we get:-
Similarly, we can prove that
Hence from equations 1 and 2 we get:-
Hence proved
Note: In this type of question students should first analyse the diagram instead of solving the equations. All the vectors should be taken according to their directions.
Also while calculating the magnitude of the vector product, students should take the correct angle between the two vectors.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

In which part of the body the blood is purified oxygenation class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




