
Prove in a triangle ABC, if
Answer
495k+ views
Hint:We start solving the problem by drawing all the given information to get a better view. We use the fact that the angle opposite to the larger side is large to get the relation between angles
Complete step by step answer:
According to the problem we have a triangle
Let us draw the given information to get a better view.
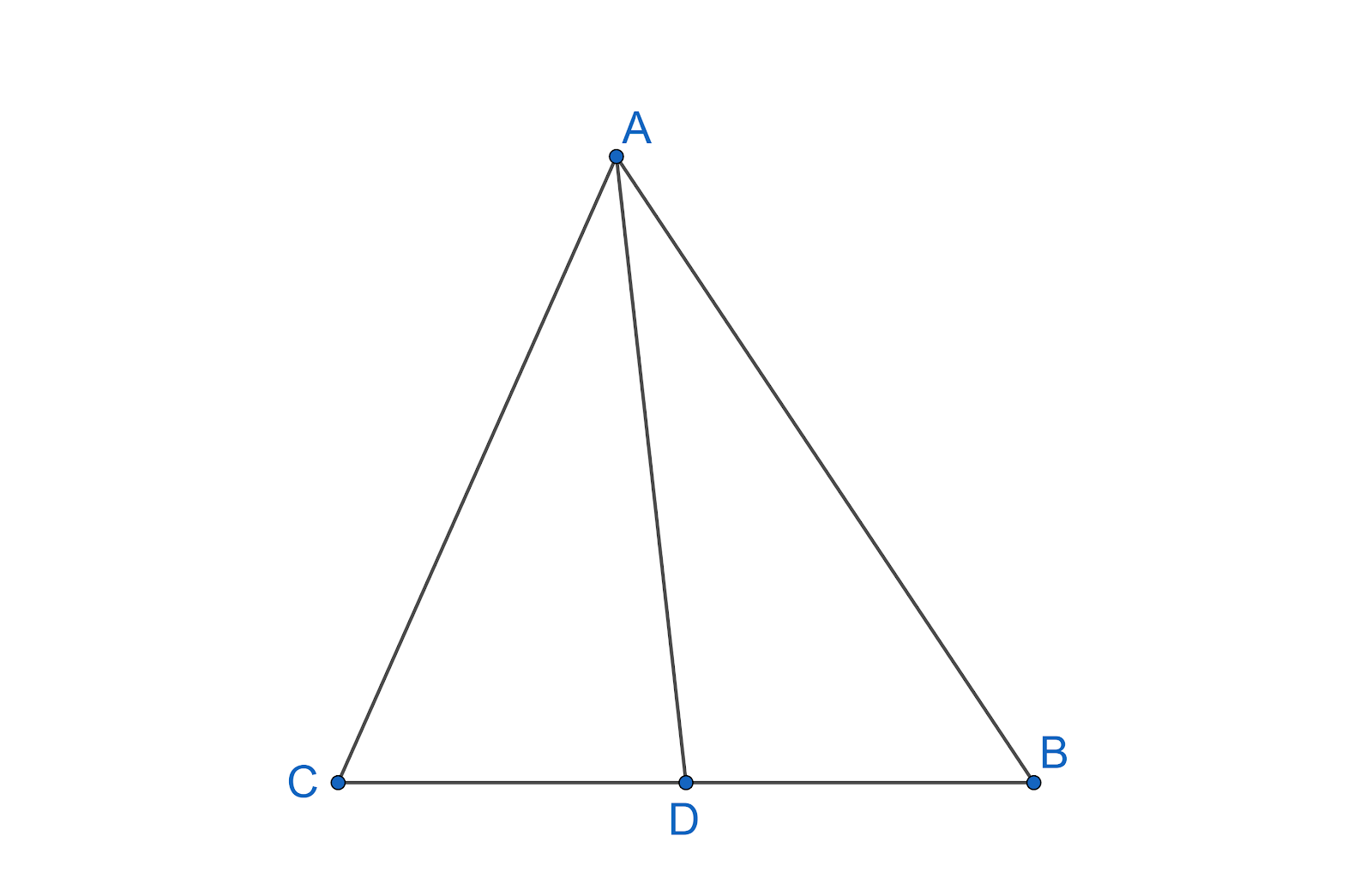
According to the problem, we have a relation between sides AB and AC as
We know that the angle in a straight line at a point is
We get
We know that the sum of the angles in a triangle is
So, we get
From equation (2), we get
From the figure, we can see that
From equation (4), we can see that the value of the angle
So, we get
From equation (1) and (5), we can clearly see that
Now, let us consider
So, we get
∴ We have proved
Note:
We should not confuse with the notation of angles present inside the triangle. Whenever we get this type of problem, we should make use of the diagram and the fact that the angle opposite to the larger side is large. We should not assume the angle at point D as
Complete step by step answer:
According to the problem we have a triangle
Let us draw the given information to get a better view.

According to the problem, we have a relation between sides AB and AC as
We know that the angle in a straight line at a point is
We get
We know that the sum of the angles in a triangle is
So, we get
From equation (2), we get
From the figure, we can see that
From equation (4), we can see that the value of the angle
So, we get
From equation (1) and (5), we can clearly see that
Now, let us consider
So, we get
∴ We have proved
Note:
We should not confuse with the notation of angles present inside the triangle. Whenever we get this type of problem, we should make use of the diagram and the fact that the angle opposite to the larger side is large. We should not assume the angle at point D as
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Chandigarh is the capital of A Punjab B Haryana C Punjab class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




